Hostname: page-component-cd9895bd7-gvvz8 Total loading time: 0 Render date: 2024-12-30T20:53:09.340Z Has data issue: false hasContentIssue false
  • English
  • Français

On the intersection of random rotations of a symmetric convex body

Published online by Cambridge University Press:  28 March 2014

SILOUANOS BRAZITIKOS  and
PANTELIS STAVRAKAKIS
SILOUANOS BRAZITIKOS
Affiliation:
Department of Mathematics, University of Athens, Panepistimioupolis 15784, Athens, Greece. e-mail: [email protected], [email protected]
PANTELIS STAVRAKAKIS
Affiliation:
Department of Mathematics, University of Athens, Panepistimioupolis 15784, Athens, Greece. e-mail: [email protected], [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Let C be a symmetric convex body of volume 1 in 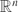${\mathbb R}^n$. We provide general estimates for the volume and the radius of C ∩ U(C) where U is a random orthogonal transformation of 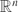${\mathbb R}^n$. In particular, we consider the case where C is in the isotropic position or C is the volume normalized Lq-centroid body Zq(μ) of an isotropic log-concave measure μ on 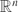${\mathbb R}^n$.

Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 157 , Issue 1 , July 2014 , pp. 13 - 30
Copyright
Copyright © Cambridge Philosophical Society 2014 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1]Borell, C.Convex measures on locally convex spaces. Ark. Mat. 12 (1974), 239–252.Google Scholar
[2]Bourgain, J.On the distribution of polynomials on high dimensional convex sets. Geom. Aspects of Funct. Analysis (Lindenstrauss-Milman eds.). Lecture Notes in Math. 1469 (1991), 127–137.Google Scholar
[3]Bourgain, J. and Milman, V. D.New volume ratio properties for convex symmetric bodies in ${\mathbb R}^n$. Invent. Math. 88 (1987), 319–340.Google Scholar
[4]Brazitikos, S., Giannopoulos, A., Valettas, P. and Vritsiou, B-H.Geometry of isotropic convex bodies. Math. Surv. Monogr. (Amer. Math. Society) 196 (2014), 594.Google Scholar
[5]Cordero–Erausquin, D., Fradelizi, M. and Maurey, B.The (B)-conjecture for the Gaussian measure of dilates of symmetric convex sets and related problems. J. Funct. Anal. 214 (2004), 410–427.Google Scholar
[6]Fleury, B.Concentration in a thin Euclidean shell for log-concave measures. J. Funct. Anal. 259 (2010), 832–841.Google Scholar
[7]Fleury, B., Guédon, O. and Paouris, G.A stability result for mean width of $Lp$-centroid bodies. Adv. Math. 214, 2 (2007), 865–877.Google Scholar
[8]Giannopoulos, A., Milman, V. D. and Tsolomitis, A.Asymptotic formulas for the diameter of sections of symmetric convex bodies. J. Funct. Anal. 223 (2005), 86–108.Google Scholar
[9]Giannopoulos, A., Stavrakakis, P., Tsolomitis, A. and Vritsiou, B-H. Geometry of the $Lq$-centroid bodies of an isotropic log-concave measure. Trans. Amer. Math. Soc. (to appear).Google Scholar
[10]Gordon, Y.On Milman's inequality and random subspaces which escape through a mesh in ${\mathbb R}^n$. Lecture Notes in Math. 1317 (1988), 84–106.Google Scholar
[11]Guédon, O. and Milman, E.Interpolating thin-shell and sharp large-deviation estimates for isotropic log-concave measures. Geom. Funct. Anal. 21 (2011), 1043–1068.Google Scholar
[12]Kashin, B. S.Sections of some finite-dimensional sets and classes of smooth functions. Izv. Akad. Nauk. SSSR Ser. Mat. 41 (1977), 334–351.Google Scholar
[13]Klartag, B.On convex perturbations with a bounded isotropic constant. Geom. Funct. Anal. 16 (2006), 1274–1290.Google Scholar
[14]Klartag, B.A central limit theorem for convex sets. Invent. Math. 168 (2007), 91–131.CrossRefGoogle Scholar
[15]Klartag, B.Power-law estimates for the central limit theorem for convex sets. J. Funct. Anal. 245 (2007), 284–310.Google Scholar
[16]Klartag, B. and Milman, E.Centroid bodies and the logarithmic Laplace transform – a unified approach. J. Fun. Anal. 262 (2012), 10–34.Google Scholar
[17]Klartag, B. and Milman, E.Inner regularization of log-concave measures and small-ball estimates. Geom. Aspects of Funct. Analysis (Klartag-Mendelson-Milman eds.), Lecture Notes in Math. 2050 (2012), 267–278.Google Scholar
[18]Klartag, B. and Vershynin, R.Small ball probability and Dvoretzky theorem, Israel J. Math. 157 (2007), 193–207.Google Scholar
[19]Latała, R. and Oleszkiewicz, K.Small ball probability estimates in terms of widths. Studia Math. 169 (2005), 305–314.Google Scholar
[20]Litvak, A. E., Pajor, A. and Tomczak–Jaegermann, N.Diameters of sections and coverings of convex bodies. J. Funct. Anal. 231 (2006), 438–457.Google Scholar
[21]Milman, V. D.Geometrical inequalities and mixed volumes in the Local Theory of Banach spaces. Astérisque 131 (1985), 373–400.Google Scholar
[22]Milman, V. D.Random subspaces of proportional dimension of finite dimensional normed spaces: approach through the isoperimetric inequality. Lecture Notes in Math. 1166 (1985), 106–115.Google Scholar
[23]Milman, V. D.Isomorphic symmetrization and geometric inequalities. Lecture Notes in Math. 1317 (1988), 107–131.Google Scholar
[24]Milman, V. D.Some applications of duality relations. Lecture Notes in Math. 1469 (1991), 13–40.Google Scholar
[25]Milman, V. D. and Schechtman, G.Asymptotic Theory of Finite Dimensional Normed Spaces. Lecture Notes in Math. 1200, (Springer, Berlin, 1986).Google Scholar
[26]Milman, V. D. and Schechtman, G.Global versus Local asymptotic theories of finite-dimensional normed spaces. Duke Math. J. 90 (1997), 73–93.Google Scholar
[27]Pajor, A. and Tomczak–Jaegermann, N.Subspaces of small codimension of finite dimensional Banach spaces. Proc. Amer. Math. Soc. 97 (1986), 637–642.Google Scholar
[28]Paouris, G.Concentration of mass in convex bodies. Geom. Funct. Anal. 16 (2006), 1021–1049.Google Scholar
[29]Paouris, G.Small ball probability estimates for log-concave measures. Trans. Amer. Math. Soc. 364 (2012), 287–308.CrossRefGoogle Scholar
[30]Pisier, G.The volume of convex bodies and banach space geometry. Cambridge Tracts in Math. 94 (1989).Google Scholar
[31]Schneider, R.Convex Bodies: The Brunn–Minkowski Theory. Encyclopedia Math. Appl. 44, (Cambridge University Press, Cambridge, 1993).Google Scholar
[32]Szarek, S. J. and Tomczak–Jaegermann, N.On nearly Euclidean decompositions of some classes of Banach spaces, Comp. Math. 40 (1980), 367–385.Google Scholar
[33]Vershynin, R.Isoperimetry of waists and local versus global asymptotic convex geometries (with an appendix by M. Rudelson and R. Vershynin). Duke Math. J. 131 (2006), 1–16.Google Scholar