Hostname: page-component-586b7cd67f-g8jcs Total loading time: 0 Render date: 2024-11-29T20:01:27.292Z Has data issue: false hasContentIssue false
  • English
  • Français

On Riemann summability of functions

Published online by Cambridge University Press:  24 October 2008

B. Kuttner
B. Kuttner
Affiliation:
University of Birmingham
Get access Rights & Permissions [Opens in a new window]

Extract

1. Let k be a positive integer. With the usual terminology, the series

will be said to be Riemann summable (R, k) to l if

converges for all sufficiently small x, and tends to l as x → 0. Here we take (sin nx)/(nx) as meaning 1 when n = 0. A more general summability method which has been considered by various authors ((1), (2), (5), (6), (8), (9), (10)), and is usually denoted by (ℜ, λ,k) is obtained by replacing (2) by

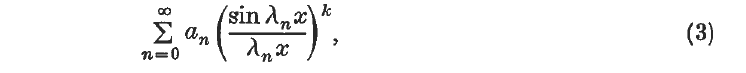
where λ = {λn} is a sequence of non-negative numbers increasing to ∞.

Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 75 , Issue 1 , January 1974 , pp. 83 - 94
Copyright
Copyright © Cambridge Philosophical Society 1974

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

(1)Burkill, H.On Riesz and Riemann summability. Proc. Cambridge Philos. Soc. 57 (1961), 55–60.CrossRefGoogle Scholar
(2)Burkill, H. and Petersen, G. M.A relation between Riesz and Riemann summability. Proc. Amer. Math. Soc. 12 (1961), 453–456.CrossRefGoogle Scholar
(3)Hardy, G. H.Divergent series. (Oxford, 1949).Google Scholar
(4)Hobson, E. W.The theory of functions of a real variable (Volume II). (Cambridge, 1926).Google Scholar
(5)Hsiang, F. C.On a theorem of Burkill–Petersen. Portugal. Math. 22 (1963), 137–141.Google Scholar
(6)Hsiang, F. C.On a theorem of Burkill. Indian J. Math. 6 (1964), 39–43.Google Scholar
(7)Kuttner, B.The relation between Riemann and Cesàro summability. Proc. London Math. Soc. (2), 38 (1935), 273–283.CrossRefGoogle Scholar
(8)Rajagopal, C. T. and Rangachari, M. S.On typical Riemann and Bessel summabilities. Proc. London Math. Soc. (3), 24 (1972), 577–589.CrossRefGoogle Scholar
(9)Rangachari, M. S.On some generalisations of Riemann summability. Math. Z. 88 (1965), 166–183, and Addendum, Math. Z. 91 (1966), 344–347.Google Scholar
(10)Russell, D. C.On Riesz and Riemann summability. Trans. Amer. Math. Soc. 104 (1962), 383–391.Google Scholar
(11)Titchmarsh, E. C.Introduction to the theory of Fourier integrals (Oxford, 1948).Google Scholar
(12)Zygmund, A.Sur la théorie riemannienne des séries trigonométriques. Math. Z. 24 (1926), 47–104.CrossRefGoogle Scholar