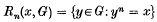Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Alekseevskii, D. V.
1985.
Lie groups.
Journal of Soviet Mathematics,
Vol. 28,
Issue. 6,
p.
924.
McCrudden, Mick
1998.
Positivity in Lie Theory.
p.
147.
Ðoković, Dragomir Ž.
and
Hofmann, Karl H.
1998.
Positivity in Lie Theory.
p.
45.
Chatterjee, Pralay
2002.
On Surjectivity of the Power Maps of Solvable Lie Groups.
Journal of Algebra,
Vol. 248,
Issue. 2,
p.
669.
Dani, S.G.
and
McCrudden, M.
2007.
Convolution roots and embeddings of probability measures on Lie groups.
Advances in Mathematics,
Vol. 209,
Issue. 1,
p.
198.
Chatterjee, Pralay
2009.
On the power maps, orders and exponentiality of p-adic algebraic groups.
Journal für die reine und angewandte Mathematik (Crelles Journal),
Vol. 2009,
Issue. 629,
Chatterjee, Pralay
2011.
Automorphism invariant Cartan subgroups and power maps of disconnected groups.
Mathematische Zeitschrift,
Vol. 269,
Issue. 1-2,
p.
221.
Chatterjee, Pralay
2011.
Surjectivity of power maps of real algebraic groups.
Advances in Mathematics,
Vol. 226,
Issue. 6,
p.
4639.
Dani, S. G.
and
Mandal, Arunava
2017.
On the surjectivity of the power maps of a class of solvable groups.
Journal of Group Theory,
Vol. 20,
Issue. 6,
p.
1089.
Gordeev, Nikolai Leonidovich
Kunyavskii, Boris Emmanuilovich
and
Plotkin, Eugene Bоrisovich
2018.
Геометрия вербальных уравнений в простых алгебраических группах над специальными полями.
Успехи математических наук,
Vol. 73,
Issue. 5(443),
p.
3.
Bhaumik, Saurav
and
Mandal, Arunava
2018.
On the density of images of the power maps in Lie groups.
Archiv der Mathematik,
Vol. 110,
Issue. 2,
p.
115.
Mandal, Arunava
2021.
Dense images of the power maps for a disconnected real algebraic group.
Journal of Group Theory,
Vol. 24,
Issue. 5,
p.
973.
Shtern, A. I.
2021.
A Version of Lie Theorem for Divisible Solvable Groups.
Russian Journal of Mathematical Physics,
Vol. 28,
Issue. 1,
p.
104.
Shtern, A. I.
2023.
Lie’s Theorem for Solvable Connected Lie Groups Without the Continuity Assumption.
Russian Journal of Mathematical Physics,
Vol. 30,
Issue. 4,
p.
701.