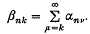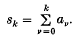Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Kangro, G. F.
1976.
Theory of summability of sequences and series.
Journal of Soviet Mathematics,
Vol. 5,
Issue. 1,
p.
1.
Maddox, I. J.
1977.
Consistency and Nörlund means.
Mathematical Proceedings of the Cambridge Philosophical Society,
Vol. 82,
Issue. 1,
p.
107.
Şengönül, Mehmet
Kayaduman, Kuddusi
and
Hirano, Norimichi
2012.
On the Riesz Almost Convergent Sequences Space.
Abstract and Applied Analysis,
Vol. 2012,
Issue. 1,
Kayaduman, Kuddusi
and
Şengönül, Mehmet
2012.
The Spaces of Cesàro Almost Convergent Sequences and Core Theorems.
Acta Mathematica Scientia,
Vol. 32,
Issue. 6,
p.
2265.
Şengönül, Mehmet
2014.
On the Zweier Sequence Spaces of Fuzzy Numbers.
International Journal of Mathematics and Mathematical Sciences,
Vol. 2014,
Issue. ,
p.
1.
Başar, Feyzi
and
Yeşilkayagil, Medine
2015.
Survey on dual summability methods.
Vol. 1676,
Issue. ,
p.
020001.
Zararsız, Zarife
2015.
Similarity Measures of Sequence of Fuzzy Numbers and Fuzzy Risk Analysis.
Advances in Mathematical Physics,
Vol. 2015,
Issue. ,
p.
1.
Raj, Kuldip
and
Sharma, Charu
2019.
A relation of Banach limit and difference matrix to generate some Orlicz sequence spaces.
Proyecciones (Antofagasta),
Vol. 38,
Issue. 4,
p.
637.