Article contents
On covering a regular polygon with a triangle
Published online by Cambridge University Press: 24 October 2008
Extract
In this note we consider a particular type of covering problem. Let T be given a plane set and Tθ be the set obtained from T by a rotation about some point in the plane through an angle θ in the clockwise sense. If a set K is such that for every θ there is a translation which transforms Tθ into a subset of K then we say that K is a rotation cover of T. The problem considered here is to determine for fixed n, l the triangle of least area which is a rotation cover for an n-sided regular polygon of side length l. In each case the solution is an equilateral triangle the altitude of which is given by
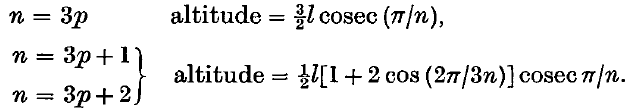
This solution is unique.
- Type
- Research Article
- Information
- Mathematical Proceedings of the Cambridge Philosophical Society , Volume 58 , Issue 1 , January 1962 , pp. 8 - 11
- Copyright
- Copyright © Cambridge Philosophical Society 1962
- 1
- Cited by


