Hostname: page-component-cd9895bd7-fscjk Total loading time: 0 Render date: 2024-12-30T19:35:14.575Z Has data issue: false hasContentIssue false
  • English
  • Français

On continuous functions with graphs of σ-finite linear measure

Published online by Cambridge University Press:  24 October 2008

James Foran
James Foran
Affiliation:
University of Wisconsin, Milwaukee, U.S.A.
Get access Rights & Permissions [Opens in a new window]

Extract

In this paper comparisons are made between the class of continuous functions of generalized bounded variation and the class of continuous functions with graphs having σ-finite length i.e. linear measure. An investigationof the differentiability and approximate differentiability of such functions discloses the fact that the latter class is considerably more extensivethan the former one. The following definitions will be needed:

(1) A function f is said to be of bounded variation (VB) on a set E if

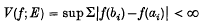
where the supremum is taken over all sequences {[ai, bi]} of non-overlapping intervals with endpoints in E.

Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 76 , Issue 1 , July 1974 , pp. 33 - 43
Copyright
Copyright © Cambridge Philosophical Society 1974

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

(1)Besicovitch, A. S. and Ursell, H. D.Sets of fractional dimension (V):On dimensional numbers of some continuous curves. J. London Math. Soc. 12 (1937), 18–25.CrossRefGoogle Scholar
(2)Garg, K. M.On level sets of a continuous nowhere monotone function. Fund. Math. 52 (1963), 59–68.CrossRefGoogle Scholar
(3)Gross, W.Über das Flächenmass von Punktmengen. Monatsch. Math. Phys. 29 (1918), 145–176.Google Scholar
(4)Saks, S.Theory of the integral, second revised edition, translated by Young, L. C. (Hafner Publishing Co.; New York, 1937).Google Scholar