Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Rao, T. S.S.R.K.
1993.
SOME REMARKS ON THE SCHUR APPROXIMATION PROPERTY.
Quaestiones Mathematicae,
Vol. 16,
Issue. 2,
p.
149.
Galbis, Antonio
1994.
Weighted Banach spaces of entire functions.
Archiv der Mathematik,
Vol. 62,
Issue. 1,
p.
58.
Castillo, Jesús M. F.
Sánchez, Fernando
and
González, Manuel
1994.
Non-Associative Algebra and Its Applications.
p.
80.
Chen, Shutao
and
Hudzik, Henryk
1995.
E Φ andh Φ fail to beM-ideals inL Φ andl Φ in the case of the Orlicz norm.
Rendiconti del Circolo Matematico di Palermo,
Vol. 44,
Issue. 2,
p.
283.
Cabello, Juan Carlos
and
Nieto, Eduardo
1998.
On Properties of $M$-Ideals.
Rocky Mountain Journal of Mathematics,
Vol. 28,
Issue. 1,
Aguirre, Francisco J
1998.
Norm-Attaining Operators into Strictly Convex Banach Spaces.
Journal of Mathematical Analysis and Applications,
Vol. 222,
Issue. 2,
p.
431.
John, Kamil
and
Werner, Dirk
2000.
M-ideals of compact operators into ℓp.
Czechoslovak Mathematical Journal,
Vol. 50,
Issue. 1,
p.
51.
Acosta, Marı́a D.
Alaminos, Jerónimo
Garcı́a, Domingo
and
Maestre, Manuel
2004.
On holomorphic functions attaining their norms.
Journal of Mathematical Analysis and Applications,
Vol. 297,
Issue. 2,
p.
625.
Acosta, María D.
and
Kim, Sung Guen
2007.
Denseness of holomorphic functions attaining their numerical radii.
Israel Journal of Mathematics,
Vol. 161,
Issue. 1,
p.
373.
Cascales, B.
Orihuela, J.
and
Galán, M. Ruiz
2013.
Computational and Analytical Mathematics.
Vol. 50,
Issue. ,
p.
161.
Angrisani, Francesca
Ascione, Giacomo
and
Manzo, Gianluigi
2019.
Orlicz spaces with a o–O type structure.
Ricerche di Matematica,
Vol. 68,
Issue. 2,
p.
841.
D’Onofrio, Luigi
Sbordone, Carlo
and
Schiattarella, Roberta
2019.
Duality and distance formulas in Banach function spaces.
Journal of Elliptic and Parabolic Equations,
Vol. 5,
Issue. 1,
p.
1.
HUANG, JINGHAO
LEVITINA, GALINA
and
SUKOCHEV, FEDOR
2020.
M-embedded symmetric operator spaces and the derivation problem.
Mathematical Proceedings of the Cambridge Philosophical Society,
Vol. 169,
Issue. 3,
p.
607.
Nessipbayev, Y.
Sukochev, F.
and
Tulenov, K.
2021.
Non-commutative analogues of weak compactness criteria in symmetric spaces.
Advances in Operator Theory,
Vol. 6,
Issue. 2,
Dantas, Sheldon
Falcó, Javier
and
Jung, Mingu
2023.
Group Invariant Operators and Some Applications to Norm-Attaining Theory.
Results in Mathematics,
Vol. 78,
Issue. 1,
Dantas, Sheldon
García-Lirola, Luis C.
Jung, Mingu
and
Zoca, Abraham Rueda
2023.
On norm-attainment in (symmetric) tensor products.
Quaestiones Mathematicae,
Vol. 46,
Issue. 2,
p.
393.


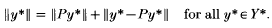
 .
.