Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Eccles, Peter John
1980.
Topology Symposium Siegen 1979.
Vol. 788,
Issue. ,
p.
23.
Eccles, Peter John
1981.
Codimension one immersions and the Kervaire invariant one problem.
Mathematical Proceedings of the Cambridge Philosophical Society,
Vol. 90,
Issue. 3,
p.
483.
Lannes, J.
1984.
Algebraic Topology Aarhus 1982.
Vol. 1051,
Issue. ,
p.
263.
Aminov, Yu. A.
1984.
Embedding problems: Geometric and topological aspects.
Journal of Soviet Mathematics,
Vol. 25,
Issue. 4,
p.
1308.
Carter, J. Scott
1986.
On generalizing Boy’s surface: constructing a generator of the third stable stem.
Transactions of the American Mathematical Society,
Vol. 298,
Issue. 1,
p.
103.
Szücs, András
1986.
Multiple points of singular maps.
Mathematical Proceedings of the Cambridge Philosophical Society,
Vol. 100,
Issue. 2,
p.
331.
Carter, J. Scott
1986.
Surgery on codimension one immersions in 𝑅ⁿ⁺¹: removing 𝑛-tuple points.
Transactions of the American Mathematical Society,
Vol. 298,
Issue. 1,
p.
83.
Carter, J. Scott
1988.
Differential Topology.
Vol. 1350,
Issue. ,
p.
144.
Szücs, András
1993.
Double point surfaces of smooth immersions Mn → ℝ2n−2.
Mathematical Proceedings of the Cambridge Philosophical Society,
Vol. 113,
Issue. 3,
p.
601.
Saeki, Osamu
1995.
Separation by a codimension-1 map with a normal crossing point.
Geometriae Dedicata,
Vol. 57,
Issue. 3,
p.
235.
Asadi-Golmankhaneh, Mohammad A
and
Eccles, Peter J
2000.
Double point self-intersection surfaces of immersions.
Geometry & Topology,
Vol. 4,
Issue. 1,
p.
149.
Asadi-Golmankhaneh, Mohammad A.
2009.
Double point self-transverse immersions of M8k↬R16k−4.
Topology and its Applications,
Vol. 156,
Issue. 15,
p.
2452.
ASADI-GOLMANKHANEH, Mohammad A.
2010.
Double point of self-transverse immersions of ${M^{2n} \looparrowright \boldsymbol{R}^{4n-5}}$.
Journal of the Mathematical Society of Japan,
Vol. 62,
Issue. 4,
Asadi-Golmankhaneh, Mohammad A.
2011.
Multiple points of immersions of M6 ↬ (ℝ7).
Acta Mathematica Scientia,
Vol. 31,
Issue. 1,
p.
259.
Akhmetʼev, Petr
and
Frolkina, Olga
2013.
On non-immersibility ofRP10toR15.
Topology and its Applications,
Vol. 160,
Issue. 11,
p.
1241.
Zare, Hadi
2018.
On the Unstable Hurewicz Homomorphism and Toda Brackets.
Bulletin of the Iranian Mathematical Society,
Vol. 44,
Issue. 3,
p.
781.
Ghasemi, Khadijeh
Janfada, Ali S.
and
Zare, Hadi
2023.
Bordism of self-intersection manifolds of certain codimension one immersions.
Boletín de la Sociedad Matemática Mexicana,
Vol. 29,
Issue. 3,


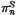 with the bordism group of oriented compact closed smooth
with the bordism group of oriented compact closed smooth