Hostname: page-component-586b7cd67f-gb8f7 Total loading time: 0 Render date: 2024-11-26T18:16:21.361Z Has data issue: false hasContentIssue false
  • English
  • Français

Linear forms in algebraic points of Abelian functions. II

Published online by Cambridge University Press:  24 October 2008

D. W. Masser
D. W. Masser
Affiliation:
(University of Nottingham)
Get access Rights & Permissions [Opens in a new window]

Extract

In this paper we continue to develop the apparatus needed for the proof of the theorem announced in (11). We retain the notation of (11) together with the assumptions made there about the field 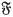of Abelian functions. This section deals with properties of more general functions holomorphic on Cn. When n = 1 the extrapolation procedure in problems of transcendence is essentially the maximum modulus principle together with the act of dividing out zeros of an analytic function. For n > 1, however, this approach is not possible, and some mild theory of several complex variables is required. This was first used in the context of transcendence by Bombieri and Lang in (2) and (12), and we now give a brief account of the basic constructions of their papers.

Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 79 , Issue 1 , January 1976 , pp. 55 - 70
Copyright
Copyright © Cambridge Philosophical Society 1976

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

(1)Baker, A.Linear forms in the logarithms of algebraic numbers. Mathematika 13 (1966), 204–16.CrossRefGoogle Scholar
(2)Bombieri, E.Algebraic values of meromorphic maps. Inventiones math. 10 (1970), 267–87.Google Scholar
(3)Cassels, J. W. S.An introduction to diophantine approximation. Cambridge Tracts no. 45. (Cambridge University Press 1957.)Google Scholar
(4)Gelfond, A. O.Transcendental and algebraic numbers (New York; Dover, 1960).Google Scholar
(5)Landau, E.Einführung in die elementare und analytische Theorie der algebraischen Zahlen und der Ideale (Leipzig; Teubner, 1918).Google Scholar
(6)Lang, S.Introduction to transcendental numbers (Reading; Addison-Wesley, 1966).Google Scholar
(7)Masser, D. W. Elliptic functions and transcendence. Ph.D. thesis, University of Cambridge, 1974. (Lecture Notes in Mathematics, 437, Springer, 1975.)Google Scholar
(8)Roth, K. F.Rational approximations to algebraic numbers. Mathematika 2 (1955), 1–20.Google Scholar
(9)Schneider, T.Einführung in die transzendenten Zahlen (Berlin; Springer-Verlag, 1957).CrossRefGoogle Scholar
(10)Siegel, C. L.Topics in Complex Function Theory, vol. III (New York; Wiley-Interscience, 1973).Google Scholar
(11)Masser, D. W.Linear forms in algebraic points of Abelian functions. I. Proc. Cambridge Philos. Soc. 77 (1975), 499–513.CrossRefGoogle Scholar
(12)Bombieri, E. and Lang, S.Analytic subgroups of group varieties. Inventiones math. 11 (1970), 1–14.Google Scholar