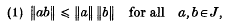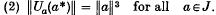Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Youngson, M. A.
1981.
Non-unital Banach Jordan algebras and C*-triple systems.
Proceedings of the Edinburgh Mathematical Society,
Vol. 24,
Issue. 1,
p.
19.
Pay�, Rafael
Perez, Javier
and
Rodr�guez, Angel
1982.
Noncommutative Jordan C*-algebras.
Manuscripta Mathematica,
Vol. 37,
Issue. 1,
p.
87.
Kaup, Wilhelm
1983.
A Riemann mapping theorem for bounded symmetric domains in complex Banach spaces.
Mathematische Zeitschrift,
Vol. 183,
Issue. 4,
p.
503.
1985.
Symmetric Banach Manifolds and Jordan C*-Algebras.
Vol. 104,
Issue. ,
p.
425.
Rodr�guez Palacios, Angel
1988.
Jordan axioms for C*-algebras.
Manuscripta Mathematica,
Vol. 61,
Issue. 3,
p.
297.
Iochum, B.
Loupias, G.
and
Rodriguez-Palacios, A.
1989.
Commutativity ofC*-algebras and associativity ofJB*-algebras.
Mathematical Proceedings of the Cambridge Philosophical Society,
Vol. 106,
Issue. 2,
p.
281.
Pérez, J.
Rico, L.
and
Rodríguez, A.
1994.
Full subalgebras of Jordan-Banach algebras and algebra norms on 𝐽𝐵*-algebras.
Proceedings of the American Mathematical Society,
Vol. 121,
Issue. 4,
p.
1133.
Moreno Galindo, Antonio
and
Rodríguez Palacios, Ángel
2010.
On multiplicatively closed subsets of normed algebras.
Journal of Algebra,
Vol. 323,
Issue. 5,
p.
1530.
Rodríguez Palacios, Ángel
and
Cabrera García, Miguel
2021.
Algebra and Applications 1.
p.
111.