Isometries of self-adjoint complex function spaces
Published online by Cambridge University Press: 24 October 2008
Extract
By a complex function space A we will mean a uniformly closed linear space of continuous complex-valued functions on a compact Hausdorff space X, such that A contains constants and separates the points of X. We denote by S the state-space
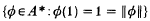
endowed with the w*-topology. If A is self-adjoint then it is well known (cf. [1]) that A is naturally isometrically isomorphic to 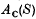 , and re A is naturally isometrically isomorphic to A(S), where
, and re A is naturally isometrically isomorphic to A(S), where 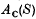 (respectively A(S)) denotes the Banach space of all complex-valued (respectively real-valued) continuous affine functions on S with the supremum norm.
(respectively A(S)) denotes the Banach space of all complex-valued (respectively real-valued) continuous affine functions on S with the supremum norm.
- Type
- Research Article
- Information
- Mathematical Proceedings of the Cambridge Philosophical Society , Volume 105 , Issue 1 , January 1989 , pp. 133 - 138
- Copyright
- Copyright © Cambridge Philosophical Society 1989
References
REFERENCES
- 1
- Cited by


