In this paper the method of infinitesimal transformations of coordinates, used by Weyl to determine conditions that a function of the tensors gik and φi, and certain of their derivatives, should be a scalar density, is applied (with certain modifications so as to give tensor relations) to functions of 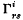and 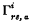. It is known that in order that such a function should be a scalar density it must be a homogeneous function, of degree ½n, of 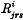, and this must of course be deducible from the equations found by the infinitesimal transformations. In view of the part which these equations may play, as “equations of energy,” etc., in purely affine field theories, it seems desirable that the connection should be explicitly shown, and this is done in § 3.