Hostname: page-component-cd9895bd7-fscjk Total loading time: 0 Render date: 2024-12-28T13:12:19.605Z Has data issue: false hasContentIssue false
  • English
  • Français

Harmonic G-structures

Published online by Cambridge University Press:  01 March 2009

J. C. GONZÁLEZ–DÁVILA  and
F. MARTÍN CABRERA
J. C. GONZÁLEZ–DÁVILA
Affiliation:
Department of Fundamental Mathematics, University of La Laguna, 38200 La Laguna, Tenerife, Spain. e-mail: [email protected], [email protected]
F. MARTÍN CABRERA
Affiliation:
Department of Fundamental Mathematics, University of La Laguna, 38200 La Laguna, Tenerife, Spain. e-mail: [email protected], [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

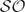For closed and connected subgroups G of SO(n), we study the energy functional on the space of G-structures of a (compact) Riemannian manifold (M, 〈⋅, ⋅〉), where G-structures are considered as sections of the quotient bundle (M)/G. We deduce the corresponding first and second variation formulae and the characterising conditions for critical points by means of tools closely related to the study of G-structures. In this direction, we show the rôle in the energy functional played by the intrinsic torsion of the G-structure. Moreover, we analyse the particular case G=U(n) for 2n-dimensional manifolds. This leads to the study of harmonic almost Hermitian manifolds and harmonic maps from M into 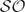(M)/U(n).

Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 146 , Issue 2 , March 2009 , pp. 435 - 459
Copyright
Copyright © Cambridge Philosophical Society 2008

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1]Besse, A. L.Einstein manifolds. Ergebnisse der Mathematik und ihrer Grenzgebiete 3. Folge 10 (Springer, 1987).CrossRefGoogle Scholar
[2]Bor, G., Hernández Lamoneda, L. and Salvai, M.Orthogonal almost-complex structures of minimal energy on conformally, and half-conformally flat manifolds. Geom. Dedicata 127 (2007), 75–85. arXiv:math.DG/0609511CrossRefGoogle Scholar
[3]Eells, J. and Lemaire, L.A report on harmonic maps. Bull. London Math. Soc. 10 (1978), 1–68.CrossRefGoogle Scholar
[4]Eells, J. and Lemaire, L.Another report on harmonic maps. Bull. London Math. Soc. 20 (1988), 385–524.CrossRefGoogle Scholar
[5]Eells, J. and Sampson, J. H.Harmonic mappings of Riemannian manifolds. Amer. J. Math. 86 (1964), 109–160.CrossRefGoogle Scholar
[6]Cleyton, R. and Swann, A. F.Einstein metrics via intrinsic or parallel torsion. Math. Z. 247 no. 3 (2004), 513–528.CrossRefGoogle Scholar
[7]Falcitelli, M., Farinola, A. and Salamon, S. M.Almost-Hermitian geometry. Differential Geom. Appl. 4 (1994), 259–282.CrossRefGoogle Scholar
[8]Gil-Medrano, O., González–Dávila, J. C. and Vanhecke, L.Harmonicity and minimality of oriented distributions. Israel J. Math. 143 (2004), 253–279.CrossRefGoogle Scholar
[9]González–Dávila, J. C., Cabrera, F. Martín and Salvai, M. Harmonicity of sections of sphere bundles. Math. Z. to appear. arXiv:math.DG/0711.3703Google Scholar
[10]Gray, A.Almost complex submanifolds of the six sphere. Proc. Amer. Math. Soc. 20 (1970), 277–279.CrossRefGoogle Scholar
[11]Gray, A.Nearly Kähler manifolds. J. Diff. Geom. 4 (1976), 283–309.Google Scholar
[12]Gray, A.Curvature identities for Hermitian and almost Hermitian manifolds. Tôhoku Math. J. (2) 28 no. 4 (1976), 601–612.CrossRefGoogle Scholar
[13]Gray, A. and Hervella, L. M.The sixteen classes of almost Hermitian manifolds and their linear invariants. Ann. Mat. Pura Appl. (4) 123 (1980), 35–58.CrossRefGoogle Scholar
[14]Kirichenko, V. F.K-spaces of maximal rank. Mat. Zametki 22 (1977), 465–476.Google Scholar
[15]Lawson, B. and Michelsohn, M. L.Spin Geometry. (Princeton University Press, 1989).Google Scholar
[16]Martín Cabrera, F. and Swann, A.Curvature of special almost Hermitian manifolds. Pacific J. Math. 228 (2006), 165–184. arXiv:math.DG/0501062CrossRefGoogle Scholar
[17]Sakai, T.Riemannian Geometry. Transl. Math. Mon. 149 (Amer. Math. Soc., 1996).Google Scholar
[18]Salamon, S.Riemannian Geometry and Holonomy Groups. Pitman Research Notes in Math. Series, 201 (Longman, 1989).Google Scholar
[19]Salvai, M.On the energy of sections of trivializable sphere bundles. Rendiconti del Seminario Matematico dell'Università e Politecnico di Torino 60 (2002), 147–155.Google Scholar
[20]Sato, T.Riemannian 3-symmetric spaces and homogeneous K-spaces. Mem. Fac. of Technology Kanazawa Univ. 12 (2) (1979), 137–143.Google Scholar
[21]Tricerri, F. and Vanhecke, L.Curvature tensors on almost Hermitian manifolds. Trans. Amer. Math. Soc. 267 (1981), 365–398.CrossRefGoogle Scholar
[22]Urakawa, H.Calculus of variations and harmonic maps. Transl. Math. Mon. 132 (Amer. Math. Soc., 1993).Google Scholar
[23]Vaisman, I.Locally conformal Kähler manifolds with parallel Lee form. Rend. Mat. (6) 12 no. 2 (1979), 263–284.Google Scholar
[24]Vilms, J.Totally geodesic maps. J. Diff. Geom. 4 (1970), 73–79.Google Scholar
[25]Wiegmink, G.Total bending of vector fields on Riemannian manifolds. Math. Ann. 303 (1995), 325–344.CrossRefGoogle Scholar
[26]Wood, C. M.Harmonic almost-complex structures. Comp. Math. 99 (1995), 183–212.Google Scholar
[27]Wood, C. M.On the energy of a unit vector field. Geom. Dedicata 64 (1997), 319–330.CrossRefGoogle Scholar
[28]Wood, C. M.Harmonic sections of homogeneous fibre bundles. Differential Geom. Appl. 19 (2003), 193–210.CrossRefGoogle Scholar