No CrossRef data available.
Gradient configurations and quadratic functions
Published online by Cambridge University Press: 24 October 2008
Extract
A normal preference system for a combination of goods is represented by an increasing function φ with convex levels. From Gossen's law, that preference and price directions coincide in equilibrium (a special consequence of his Second Law), it follows that, on the data that xr is the vector of quantities purchased at prices given by a vector pr (r = 1,…, k), the gradient gr = g(xr) of the function φ at the point xr is given by
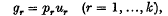
for some positive multiplier μr;. There may be considered the class of preference functions thus satisfying Gossen's law in respect to the data, and thus with gradients taking prescribed directions at k prescribed points. In particular, there may be considered the subclass of these which are quadratic in some convex region containing the points xr. By choosing any multipliers μr, there is obtained a set of gradients gr associated with the points xr. It is asked if there exists a quadratic function which is increasing and has convex levels in a convex neighbourhood of the points xr, and whose gradient at xr is gr; also it is required to characterize the class of such functions, which, if any exist, form an infinite variety. This is the background of the questions which are going to be investigated, and which are of importance in a general method of empirical preference analysis in economics.
- Type
- Research Article
- Information
- Mathematical Proceedings of the Cambridge Philosophical Society , Volume 59 , Issue 2 , April 1963 , pp. 287 - 305
- Copyright
- Copyright © Cambridge Philosophical Society 1963
References
† Gossen, H.Entwickelung der Gesetze des Menschlichen Verhehrs, und der darans fliessenden Regeln für menschliches Handeln (Braunschweig, 1854).Google Scholar
‡ Afriat, S. N., Preference analysis: a general method with application to the cost of living index. Research Memorandum, no. 29. Econometric Research Program, Princeton University, 08 1961.Google Scholar


