Hostname: page-component-586b7cd67f-t7czq Total loading time: 0 Render date: 2024-11-26T07:41:17.294Z Has data issue: false hasContentIssue false
  • English
  • Français

A family of Fitting classes of supersoluble groups

Published online by Cambridge University Press:  24 October 2008

Martin Menth
Martin Menth
Affiliation:
Universität Würzburg, Mathematisches Institut, Am Hubland, D-97074 Würzburg, Germany
Get access Rights & Permissions [Opens in a new window]

Extract

A class of groups that is closed with respect to subnormal subgroups and normal products is called a Fitting class. Given a finite soluble group G, one may ask for the Fitting class 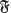(G) generated by G, that is the intersection of all Fitting classes containing G. For simple or nilpotent groups G it is easy to compute 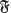(G), but in other cases the determination of 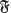(G) seems to be surprisingly difficult, and there is no general method of solving this problem. In recent years there has been a lot of work in this area, see for instance Bryce and Cossey[l], [2], Hawkes[6] (or [5], IX. 9. Var. II), Heineken[7] and McCann[10].

Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 118 , Issue 1 , July 1995 , pp. 49 - 57
Copyright
Copyright © Cambridge Philosophical Society 1995

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1]Bryce, R. A.. The Fitting class generated by a finite soluble group. Ann. Mat. Pura Appl. (4) 159 (1991), 151–169.Google Scholar
[2]Bryce, R. A. and Cossey, J.. Fitting classes after Dark. Group Theory Singapore 1987, 293–321 (deGruyter, 1989).Google Scholar
[3]Dark, R.. Some examples in the theory of injectors of finite soluble groups. Math. Z. 127 (1972), 145–156.CrossRefGoogle Scholar
[4]Dark, R.. A complete group of odd order. Math. Proc. Cambridge Phil. Soc. 77 (1975), 21–28.CrossRefGoogle Scholar
[5]Doerk, K. and Hawkes, T.. Finite soluble groups (deGruyter, 1992).CrossRefGoogle Scholar
[6]Hawkes, T.. On metanilpotent Fitting classes. J. Algebra 63 (1980), 459–483.CrossRefGoogle Scholar
[7]Heineken, H.. Fitting classes of certain metanilpotent groups. Glasgow Math. J. 36 (1994), 185–195.CrossRefGoogle Scholar
[8]Huppert, B.. Monomiale Darstellung endlicher Gruppen. Nagoya Math. J. 6 (1953), 93–94.CrossRefGoogle Scholar
[9]lockett, F. P.. On the theory of Fitting classes of finite soluble groups. Ph.D. thesis, University of Warwick (1971).Google Scholar
[10]McCann, B.. On Fitting classes of groups of nilpotent length three. Ph.D. thesis, University of Würzburg (1985).Google Scholar
[11]Robinson, D. J. S.. A course in the theory of groups (Springer, 1982).CrossRefGoogle Scholar