Published online by Cambridge University Press: 14 February 2023
Let
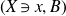 $(X\ni x,B)$
be an lc surface germ. If
$(X\ni x,B)$
be an lc surface germ. If
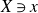 $X\ni x$
is klt, we show that there exists a divisor computing the minimal log discrepancy of
$X\ni x$
is klt, we show that there exists a divisor computing the minimal log discrepancy of
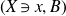 $(X\ni x,B)$
that is a Kollár component of
$(X\ni x,B)$
that is a Kollár component of
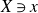 $X\ni x$
. If
$X\ni x$
. If
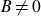 $B\not=0$
or
$B\not=0$
or
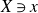 $X\ni x$
is not Du Val, we show that any divisor computing the minimal log discrepancy of
$X\ni x$
is not Du Val, we show that any divisor computing the minimal log discrepancy of
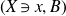 $(X\ni x,B)$
is a potential lc place of
$(X\ni x,B)$
is a potential lc place of
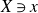 $X\ni x$
. This extends a result of Blum and Kawakita who independently showed that any divisor computing the minimal log discrepancy on a smooth surface is a potential lc place.
$X\ni x$
. This extends a result of Blum and Kawakita who independently showed that any divisor computing the minimal log discrepancy on a smooth surface is a potential lc place.