One method of measuring frequency depends upon resonance. If a body of unit mass, subject to elastic constraints and experiencing a damping resistance proportional to its speed, be acted on by a periodic force F sin pt, the equation of motion may be written

The amplitude of the steady vibration ultimately set up is

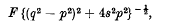
the maximum value occurring when p2 = q2 − 2s2. If the motion, in the absence of the dri ving force F sin pt, be only slightly damped, s2 is very small compared with q2, and little error will be made if the value of p giving maximum amplitude be taken as equal to q. When s/q is small, the resonance is “sharp,” i.e. a small discrepancy between p and q, or, more strictly, between p and (q2 − 2s2)½, causes the amphtude to fall far below its maxìmum value. Thus, when the damping is small, maximum amplitude, f or variation of p, indicates that p is very nearly equal to q.