Hostname: page-component-cd9895bd7-mkpzs Total loading time: 0 Render date: 2024-12-28T14:06:37.007Z Has data issue: false hasContentIssue false
  • English
  • Français

Contributions to the theory of Ramanujan's function τ(n) and similar arithmetical functions

II. The order of the Fourier coefficients of integral modular forms

Published online by Cambridge University Press:  24 October 2008

R. A. Rankin
R. A. Rankin
Affiliation:
Clare CollegeCambridge
Get access Rights & Permissions [Opens in a new window]

Extract

Suppose that

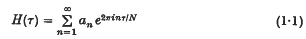
is an integral modular form of dimensions −κ, where κ > 0, and Stufe N, which vanishes at all the rational cusps of the fundamental region, and which is absolutely convergent for 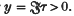Then

where a, b, c, d are integers such that ad − bc = 1.

Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 35 , Issue 3 , July 1939 , pp. 357 - 372
Copyright
Copyright © Cambridge Philosophical Society 1939

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

* Cf. for example, Klein, F. and Fricke, R., Elliptische Modulfunktionen, 1 (Leipzig, 1890), 395–7.Google Scholar

* Salié, H., “Zur Abschätzung der Fourierkoeffizienten ganzer Modulformen”, Math. Z. 36 (1931), 263–78.CrossRefGoogle Scholar

† Davenport, H., “On certain exponential sums”, J. reine angew. Math. 169 (1932), 158–76.Google Scholar

‡ Hauptkongruenzgruppe.

* Cf. Landau, E., Primzahlen, 1 (Leipzig, 1909), 483–92.Google Scholar

† This may be proved in several ways; cf., for example Hecke, E., “Über Modulfunktionen und die Dirichletschen Reihen mit Eulerscher Produktentwicklung I”, Math. Ann. 114 (1937), 1–28 (Satz 5).CrossRefGoogle Scholar

* Cf. for example E. Hecke, loc. cit., Satz 7.

† By the Wiener-Ikehara theorem we can deduce at once from Theorem 3 that

See Bochner, S., “Ein Satz von Landau und Ikehara”, Math. Z. 37 (1933), 1–9.CrossRefGoogle Scholar

‡ Landau, E., “Über die Anzahl der Gitterpunkte in gewissen Bereichen. II”, Nachr. Ges. Wiss. Göttingen (1915), pp. 209–43.Google Scholar

* | αγ, δ (n)| is not dependent on α, β.

† When (m, n)>1, (m, n, N) = 1, we denote by f m n (s) the function f γ,δ(s), where γ ≡ m, δ ≡ n (mod N), (γ, δ) = 1.

* This is trivial when It is true also for since Landau's theorem can be extended to show that

for any real α > − β, where R(a, x) is the sum of the residues of in the strip

I write k′ where Landau has k to avoid confusion with the dimension − k.