Hostname: page-component-586b7cd67f-g8jcs Total loading time: 0 Render date: 2024-11-20T14:20:58.842Z Has data issue: false hasContentIssue false
  • English
  • Français

Constructive complete distributivity. I

Published online by Cambridge University Press:  24 October 2008

Barry Fawcett  and
R. J. Wood
Barry Fawcett
Affiliation:
Department of Mathematics, Statistics and Computing Science, Dalhousie University, Halifax, Canada
R. J. Wood
Affiliation:
Department of Mathematics, Statistics and Computing Science, Dalhousie University, Halifax, Canada
Get access Rights & Permissions [Opens in a new window]

Extract

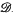The relationships, in many cases equivalences, between lattice distributivity, adjunction and continuity have been studied by many authors, for example [1, 3–8, 12, 13, 15, 17–20, 22, 23]. Very roughly, we refer to the following circle of ideas. Let L be an ordered set, and L a class of subsets of L, and suppose that L has a supremum for each element in 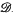L. We might say that L has 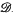-sups. The ‘distributivity’ we refer to is that of infs over 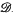-sups. The ‘adjunction’ is that given by a left adjoint to the map V: 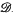L→L. Now the latter has a left adjoint if and only if it preserves infs, and this means roughly that the 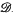-sup of an intersection is an inf of 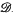-sups. When one does succeed in identifying the 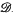-sup of an intersection as a 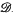-sup of infs, one has an instance of distributivity.

Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 107 , Issue 1 , January 1990 , pp. 81 - 89
Copyright
Copyright © Cambridge Philosophical Society 1990

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1]Bandelt, H.-J. and Erné, M.. The category of Z-continuous posets. J. Pure Appl. Algebra 30 (1983), 219–226.CrossRefGoogle Scholar
[2]Barr, M.. Toposes Triples and Theories (Springer-Verlag, 1985).CrossRefGoogle Scholar
[3]Bruns, G.. Distributivität und subdirekte Zerlegbarkeit vollständiger Verbände. Arch. Math. (Basel) 12 (1961), 61–66.CrossRefGoogle Scholar
[4]Bruns, G.. Darstellungen und Erweiterungen geordnete Mengen. I. J. Reine Angew. Math. 209 (1962), 167–200.CrossRefGoogle Scholar
[5]Bruns, G.. Darstellungen und Erweiterungen geordnete Mengen. II. J. Reine Angew. Math. 210 (1962), 1–23.CrossRefGoogle Scholar
[6]Büchi, J. R.. Die Boolesche Partialordnung und die Paarung von Gefügen. Portugal Math. 7 (1948), 119–190.Google Scholar
[7]Büchi, J. R.. Representation of complete lattices by sets. Portugal Math. 2 (1952), 149–186.Google Scholar
[8]Day, A.. Filter monads, continuous lattices and closure systems. Canad. J. Math. 27 (1975), 50–59.CrossRefGoogle Scholar
[9]Diaconescu, R.. Axiom of choice and complementation. Proc. Amer. Math. Soc. 51 (1975), 176–178.CrossRefGoogle Scholar
[10]Dugundji, J.. Topology (Allyn & Bacon Inc. Publishers, 1966).Google Scholar
[11]Fawcett, B. and Wood, R. J.. Constructive complete distributivity. II. (In preparation.)Google Scholar
[12]Hoffman, R.-E.. Continuous posets and adjoint sequences. Semigroup Forum 18 (1979), 173–188.CrossRefGoogle Scholar
[13]Johnstone, P. T.. Stone Spaces (Cambridge University Press, 1982).Google Scholar
[14]Kock, A. and Wraith, G. C.. Elementary toposes. Aarhus Universitet Lecture Series no. 30 (1971).Google Scholar
[15]Lawson, J. D.. The duality of continuous posets. Houston J. Math. 5 (1979), 357–386.Google Scholar
[16]Mikkelsen, C. J.. Lattice theoretic and logical aspects of elementary topoi. Aarhus Universitet Publ. Series no. 25 (1976).Google Scholar
[17]Novak, D.. Generalization of continuous posets. Trans. Amer. Math. Soc. 272 (1982), 645–667.CrossRefGoogle Scholar
[18]Raney, G. N.. Completely distributive complete lattices. Proc. Amer. Math. Soc. 3 (1952), 677–680.CrossRefGoogle Scholar
[19]Raney, G. N.. A subdirect-union representation for completely distributive lattices. Proc. Amer. Math. Soc. 4 (1953), 518–522.CrossRefGoogle Scholar
[20]Raney, G. N.. Tight Galois connections and complete distributivity. Trans. Amer. Math. Soc. 97 (1960), 418–426.CrossRefGoogle Scholar
[21]Rubin, H. and Rubin, J.. Equivalents of the Axiom of Choice (North Holland Publishing Company, 1963).Google Scholar
[22]Sikorski, R.. Representation and distributivity of Boolean algebras. Colloq. Math. 8 (1961), 1–13.CrossRefGoogle Scholar
[23]Sikorski, R.. Boolean Algebras (Academic Press, 1964).Google Scholar
[24]Street, R. and Walters, R. F. C.. Yoneda structures on 2-categories. J. Algebra 50 (1978), 350–379.CrossRefGoogle Scholar