Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Asperti, A. C.
Chaves, R. M. B.
and
Sousa, L. A. M.
2011.
The Gauss–Kronecker curvature of minimal hypersurfaces in four-dimensional space forms.
Mathematische Zeitschrift,
Vol. 267,
Issue. 3-4,
p.
523.
Savas-Halilaj, Andreas
2013.
On Deformable Minimal Hypersurfaces in Space Forms.
Journal of Geometric Analysis,
Vol. 23,
Issue. 3,
p.
1032.
Diniz, M. M.
Vilhena, J. A. M.
and
Zapata, J. F. Z.
2018.
Minimal hypersurfaces in $ {\mathbb{S}}^5$ with vanishing Gauss–Kronecker curvature.
Izvestiya: Mathematics,
Vol. 82,
Issue. 3,
p.
477.
Diniz, Marcos Monteiro
Vilhena, Jose Antonio Moraes
and
Zapata, Juan Fernando Zapata
2018.
Минимальные гиперповерхности в $\mathbb{S}^5$ с нулевой кривизной Гаусса-Кронекера.
Известия Российской академии наук. Серия математическая,
Vol. 82,
Issue. 3,
p.
31.


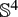 with zero Gauss–Kronecker curvature
with zero Gauss–Kronecker curvature