Hostname: page-component-cd9895bd7-lnqnp Total loading time: 0 Render date: 2024-12-28T13:20:48.588Z Has data issue: false hasContentIssue false
  • English
  • Français

Complete Bredon cohomology and its applications to hierarchically defined groups

Published online by Cambridge University Press:  08 April 2016

BRITA E. A. NUCINKIS  and
NANSEN PETROSYAN
BRITA E. A. NUCINKIS
Affiliation:
Department of Mathematics, Royal Holloway, University of London, Egham, TW20 0EX. e-mail: [email protected]
NANSEN PETROSYAN
Affiliation:
School of Mathematics, University of Southampton, Southampton SO17 1BJ. e-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

By considering the Bredon analogue of complete cohomology of a group, we show that every group in the class 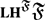$\cll\clh^{\mathfrak F}{\mathfrak F}$ of type Bredon-FP∞ admits a finite dimensional model for $E_{\frak F}G$.

We also show that abelian-by-infinite cyclic groups admit a 3-dimensional model for the classifying space for the family of virtually nilpotent subgroups. This allows us to prove that for 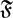$\mathfrak {F}$, the class of virtually cyclic groups, the class of 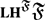$\cll\clh^{\mathfrak F}{\mathfrak F}$-groups contains all locally virtually soluble groups and all linear groups over ${\mathbb{C}}$ of integral characteristic.

Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 161 , Issue 1 , July 2016 , pp. 143 - 156
Copyright
Copyright © Cambridge Philosophical Society 2016 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1]Alperin, R. C. and Shalen, P. B.Linear groups of finite cohomological dimension. Invent. Math 66 (1982), 89–98.Google Scholar
[2]Benson, D. J. and Carlson, J. F.Products in negative cohomology. J. Pure Appl. Algebra 82 (1992), no. 2, 107–129.Google Scholar
[3]Bredon, G. E.Equivariant cohomology theories. Lecture Notes in Mathematics, No. 34 (Springer-Verlag, Berlin, 1967), MR0214062 (35 #4914)Google Scholar
[4]Degrijse, D., Köhl, R. and Petrosyan, N. Classifying spaces with virtually cyclic stabilizers for linear groups, preprint in preparation.Google Scholar
[5]Degrijse, D. and Petrosyan, N.Commensurators and classifying spaces with virtually cyclic stabilizers. Groups Geom. Dyn. 7 (2013), no. 3, 543–555.Google Scholar
[6]Degrijse, D. and Petrosyan, N.Geometric dimension of groups for the family of virtually cyclic subgroups. J. Topol. 7 (2014), no. 3, 697–726.Google Scholar
[7]Degrijse, D. and Petrosyan, N.Bredon cohomological dimensions for groups acting on CAT(0)-spaces. Groups Geom. Dyn. 9 (2015), no. 4, 1231–1265.Google Scholar
[8]Dembegioti, F., Petrosyan, N. and Talelli, O.Intermediaries in Bredon (co)homology and classifying spaces. Publ. Mat. 56 (2012), no. 2, 393–412.Google Scholar
[9]Flores, R. J. and Nucinkis, B. E. A.On Bredon homology of elementary amenable groups. Proc. Amer. Math. Soc. 135 (2005), no. 1, 5–11 (electronic). MR2280168.Google Scholar
[10]Fluch, M.Classifying spaces with virtually cyclic stabilisers for certain infinite cyclic extensions, J. Pure Appl. Algebra 215 (2011), no. 10, 2423–2430.Google Scholar
[11]Fluch, M. G. and Nucinkis, B. E. A.On the classifying space for the family of virtually cyclic subgroups for elementary amenable groups. Proc. Amer. Math. Soc. 141 (2013), no. 11, 3755–3769.Google Scholar
[12]Juan-Pineda, D. and Leary, I. J.. On classifying spaces for the family of virtually cyclic subgroups. Recent developments in algebraic topology. (2006), pp. 135–145. MR2248975 (2007d:19001)Google Scholar
[13]Kochloukova, D. H., Martínez–Pérez, C. and Nucinkis, B. E. A.. Cohomological finiteness conditions in Bredon cohomology. Bull. Lond. Math. Soc. 43 (2011), no. 1, 124–136.CrossRefGoogle Scholar
[14]Kropholler, P. H., Martínez-Pérez, C. and Nucinkis, B. E. A.. Cohomological finiteness conditions for elementary amenable groups. J. Reine Angew. Math. 637 (2009), 49–62. MR2599081Google Scholar
[15]Kropholler, P. H.On groups of type (FP)∞. J. Pure Appl. Algebra. 90 (1993), no. 1, 55–67. MR1246274 (94j:20051bGoogle Scholar
[16]Kropholler, P. H. On groups with many finitary cohomology functors. preprint (2013).Google Scholar
[17]Lafont, J.-F. and Ortiz, I. J.. Relative hyperbolicity, classifying spaces and lower algebraic K-theory. Topology 46 (2007), no. 6, 527–553. MR2363244Google Scholar
[18]Leary, I. J. and Nucinkis, B. E. A.Some groups of type VF. Invent. Math. 151 (2003), no. 1, 135–165.CrossRefGoogle Scholar
[19]Lück, W.Transformation groups and algebraic K-theory.Lecture Notes in Math. vol. 1408, (Springer-Verlag, Berlin, 1989) Mathematica Gottingensis. MR1027600 (91g:57036)Google Scholar
[20]Lück, W.The type of the classifying space for a family of subgroups. J. Pure Appl. Algebra 149 (2000), no. 2, 177–203. MR1757730 (2001i:55018)Google Scholar
[21]Lück, W. Survey on classifying spaces for families of subgroups. Infinite groups: Geometric, Combinatorial and Dynamical Aspects (2005), pp. 269–322. MR2195456 (2006m:55036)Google Scholar
[22]Lück, W.On the classifying space of the family of virtually cyclic subgroups for CAT(0)-groups. Münster J. Math. 2 (2009), 201–214. MR2545612 (2011a:20107)Google Scholar
[23]Lück, W. and Meintrup, D.On the universal space for group actions with compact isotropy. Geometry and topology (Aarhus, 1998), 2000, pp. 293–305. MR1778113 (2001e:55023)Google Scholar
[24]Lück, W. and Weiermann, M.On the classifying space of the family of virtually cyclic subgroups. Pure App. Math. Q. 8 (2012), no. 2, 479–555.Google Scholar
[25]Mac Lane, S.Homology. Classics in Mathematics (Springer-Verlag, Berlin, (1995). Reprint of the 1975 edition.Google Scholar
[26]Mac Lane, S.Categories for the working mathematician. Second Ed., Graduate Texts in Math. vol. 5 (Springer-Verlag, New York, (1998), MR1712872 (2001j:18001)Google Scholar
[27]Martinez-Pérez, C. and Nucinkis, B. E. A.. Bredon cohomological finiteness conditions for generalisations of Thompson groups. Groups, Geometry, Dynamics 7 (2013), 931–959.Google Scholar
[28]Meintrup, D. and Schick, T.A model for the universal space for proper actions of a hyperbolic group. New York J. Math. 8 (2002), 1–7 (electronic)Google Scholar
[29]Mislin, G.Tate cohomology for arbitrary groups via satellites. Topology Appl. 56 (1994), no. 3, 293–300.Google Scholar
[30]Symonds, P.The Bredon cohomology of subgroup complexes. J. Pure Appl. Algebra 199 (2005), no. 3, 261–298. MR2134305 (2006e:20093)Google Scholar
[31]Vogtmann, K.Automorphisms of free groups and outer space. Geom. Dedicata 94 (2002), 1–31.Google Scholar