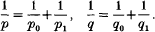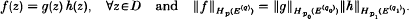Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Lust-Piquard, Françoise
1992.
A grothendieck factorization theorem on 2-convex schatten spaces.
Israel Journal of Mathematics,
Vol. 79,
Issue. 2-3,
p.
331.
Xu, Quan Hua
1992.
Radon-Nikodým property in symmetric spaces of measurable operators.
Proceedings of the American Mathematical Society,
Vol. 115,
Issue. 2,
p.
329.
Dodds, P. G.
Dodds, T. K.
Dowling, P. N.
Lennard, C. J.
and
Sukochev, F. A.
1995.
A uniform Kadec-Klee property for symmetric operator spaces.
Mathematical Proceedings of the Cambridge Philosophical Society,
Vol. 118,
Issue. 3,
p.
487.
Dodds, Peter G.
and
Dodds, Theresa K.-Y.
1995.
SOME ASPECTS OF THE THEORY OF SYMMETRIC OPERATOR SPACES.
Quaestiones Mathematicae,
Vol. 18,
Issue. 1-3,
p.
47.
Dodds, Peter G.
and
Dodds, Theresa K.
1995.
Operator Theory in Function Spaces and Banach Lattices.
p.
113.
Randrianantoanina, Narcisse
1997.
Pelczynski’s property (V*) for symmetric operator spaces.
Proceedings of the American Mathematical Society,
Vol. 125,
Issue. 3,
p.
801.
Randrianantoanina, Narcisse
1998.
Hilbert transform associated with finite maximal subdiagonal algebras.
Journal of the Australian Mathematical Society. Series A. Pure Mathematics and Statistics,
Vol. 65,
Issue. 3,
p.
388.
Randrianantoanina, Narcisse
2002.
Non-Commutative Martingale Transforms.
Journal of Functional Analysis,
Vol. 194,
Issue. 1,
p.
181.
Randrianantoanina, Narcisse
2003.
Embeddings of ℓp into Non-commutative Spaces.
Journal of the Australian Mathematical Society,
Vol. 74,
Issue. 3,
p.
331.
Pisier, Gilles
and
Xu, Quanhua
2003.
Vol. 2,
Issue. ,
p.
1459.
Randrianantoanina, Narcisse
2004.
Square function inequalities for non-commutative martingales.
Israel Journal of Mathematics,
Vol. 140,
Issue. 1,
p.
333.
Bekjan, Turdebek N.
2006.
$\Phi-Inequalities of Noncommutative Martingales.
Rocky Mountain Journal of Mathematics,
Vol. 36,
Issue. 2,
Lee, Han Ju
2007.
Complex convexity and monotonicity in quasi-Banach lattices.
Israel Journal of Mathematics,
Vol. 159,
Issue. 1,
p.
57.
Lust-Piquard, Françoise
and
Xu, Quanhua
2007.
The little Grothendieck theorem and Khintchine inequalities for symmetric spaces of measurable operators.
Journal of Functional Analysis,
Vol. 244,
Issue. 2,
p.
488.
Randrianantoanina, Narcisse
2008.
Embeddings of non-commutative L p -spaces into preduals of finite von Neumann algebras.
Israel Journal of Mathematics,
Vol. 163,
Issue. 1,
p.
1.
Kalton, N. J.
and
Sukochev, F. A.
2008.
Symmetric norms and spaces of operators.
Journal für die reine und angewandte Mathematik (Crelles Journal),
Vol. 2008,
Issue. 621,
Parcet, Javier
2009.
Pseudo-localization of singular integrals and noncommutative Calderón–Zygmund theory.
Journal of Functional Analysis,
Vol. 256,
Issue. 2,
p.
509.
Mei, Tao
and
Parcet, Javier
2009.
Pseudo-Localization of Singular Integrals and Noncommutative Littlewood–Paley Inequalities.
International Mathematics Research Notices,
Vol. 2009,
Issue. 8,
p.
1433.
Bekjan, Turdebek N.
and
Chen, Zeqian
2012.
Interpolation and Φ-moment inequalities of noncommutative martingales.
Probability Theory and Related Fields,
Vol. 152,
Issue. 1-2,
p.
179.
Czerwińska, M.M.
2012.
Complex uniform rotundity in symmetric spaces of measurable operators.
Journal of Mathematical Analysis and Applications,
Vol. 395,
Issue. 2,
p.
501.