Hostname: page-component-586b7cd67f-rcrh6 Total loading time: 0 Render date: 2024-11-26T08:53:06.305Z Has data issue: false hasContentIssue false
  • English
  • Français

The Temperature Dependence of the Photo-Electric Effect

Published online by Cambridge University Press:  24 October 2008

K. Mitchell
K. Mitchell
Affiliation:
Peterhouse; The University, Leeds
Get access Rights & Permissions [Opens in a new window]

Extract

The temperature dependence of the photo-electric effect with the image field is discussed, the result obtained agreeing with Fowler's T2 law near the threshold. Fowler's 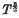law is discussed, and shown to rest upon a mistake in the normalization of the free wave functions.

The two methods of analysing experimental results due to Fowler and Du Bridge are also discussed, and it is shown that in general the latter should give the more reliable results.

The author wishes to repeat his thanks to Prof. R. H. Fowler and to Mr A. H. Wilson for their constant interest in the work.

Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 31 , Issue 3 , July 1935 , pp. 416 - 428
Copyright
Copyright © Cambridge Philosophical Society 1935

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

page 416 note * Mitchell, K., Proc. Roy. Soc. A, 146 (1934), 442CrossRefGoogle Scholar; referred to as I throughout this paper.

page 416 note † Ig. Tamm, and Schubin, S., Zeits. Physik, 68 (1931), 97CrossRefGoogle Scholar; Fröhlich, H., Ann. Physik, 7 (1930), 103CrossRefGoogle Scholar; Wentzel, G., Sommerfeld Festschrift Probleme der Modernen Physik, edited by P., Debye, p. 79 (Hirzel, 1928).Google Scholar

page 416 note ‡ Fowler, R. H., Phys. Rev. 38 (1931), 45.CrossRefGoogle Scholar

page 416 note § Young, L. A. and Frank, N. H., Phys. Rev. 38 (1931), 838.CrossRefGoogle Scholar

page 416 note ∥ Blochinzew, D., Phys. Z. Sowjetunion, 1 (1931), 781.Google Scholar

page 417 note * Bridge, L. A. Du, Phys. Rev. 39 (1932), 108.Google Scholar

page 418 note * Whittaker and Watson, Modern Analysis, Chap. xvi. Further references to this will be given in the form WW.

page 424 note † Nordheim, L. W., Proc. Roy. Soc. A, 121 (1928), 626.CrossRefGoogle Scholar

page 425 note * This is not expressly stated in his paper, but he has made it clear in conversations with the author.

page 427 note * Compare the case of the simple potential discontinuity, discussed in I. Without giving the full result for this case, we may note that the final formula will contain a power of ν, as well as a complicated function of the optical constants.

page 427 note † Bridge, L. A. Du, Phys. Rev. 39 (1932), 108.Google Scholar