The surfaces whose prime-sections contain a 
Published online by Cambridge University Press: 24 October 2008
Extract
The surfaces whose prime-sections are hyperelliptic curves of genus p have been classified by G. Castelnuovo. If p > 1, they are the surfaces which contain a (rational) pencil of conics, which traces the 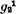 on the prime-sections. Thus, if we exclude ruled surfaces, they are rational surfaces. The supernormal surfaces are of order 4p + 4 and lie in space [3p + 5]. The minimum directrix curve to the pencil of conics—that is, the curve of minimum order which meets each conic in one point—may be of any order k, where 0 ≤ k ≤ p + 1. The prime-sections of these surfaces are conveniently represented on the normal rational ruled surfaces, either by quadric sections, or by quadric sections residual to a generator, according as k is even or odd.
on the prime-sections. Thus, if we exclude ruled surfaces, they are rational surfaces. The supernormal surfaces are of order 4p + 4 and lie in space [3p + 5]. The minimum directrix curve to the pencil of conics—that is, the curve of minimum order which meets each conic in one point—may be of any order k, where 0 ≤ k ≤ p + 1. The prime-sections of these surfaces are conveniently represented on the normal rational ruled surfaces, either by quadric sections, or by quadric sections residual to a generator, according as k is even or odd.
- Type
- Research Article
- Information
- Mathematical Proceedings of the Cambridge Philosophical Society , Volume 30 , Issue 2 , 30 April 1934 , pp. 170 - 177
- Copyright
- Copyright © Cambridge Philosophical Society 1934
References
* Castelnuovo, G., Rend. di Palermo, 4 (1890), 73.CrossRefGoogle Scholar
* If υ is a prime number, then a pencil of elliptic curves of order υ on a rational surface is represented in the plane, either by a pencil R of cubics, or by a Halphen pencil of curves of order 3υ with nine υ-ple base-points (not in general position). In the present case, however, υ = 3, it is easily shown that the Halphen pencil gives rise to surfaces having a unode: they are in fact surfaces discussed by P. Du Val, Proc. London Math. Soc. (2), 35 (1933), 1. Hence the only non-singular surfaces F′ arise from the pencil R of cubics given in the text.
* Bronowski, J., Proc. Camb. Phil. Soc., 29 (1933), 482–3.Google Scholar
† Segre, C., Atti Ace. Torino, 21 (1885), 95.Google Scholar
* Palatini, F., Giorn. di mat., 38 (1900), 315.Google Scholar
* Enriques, F., Rend. Lincei (5), 23 (1914), 206, 291.Google Scholar
* The interesting surfaces F which have b = 1 have been discussed by Val, P. Du, Rend. Lincei (6), 15 (1932), 276.Google Scholar
- 2
- Cited by


