Some renewal theorems for random walks in multidimensional time
Published online by Cambridge University Press: 24 October 2008
Extract
Let Kr denote the set of r-tuples n = (n1n2, …, nr) with positive integers as coordinates (r ≥ 1) and {X, Xn, n ε Kr} be a family of independent, identically distributed random variables with positive mean 0 < EX ≡ μ < ∞ and finite positive variance 0 < var X ≡ σ2 ∞. The notation m ≤ n, where m = (mi) and n = (ni), means that mi ≤ ni (i = 1, 2,…, r) and |n| = n1n2 … nr. Denote Sn =Σj ≤ nXj (j, n ε Kr). When r = 1, {Xn, n ε Kr) reduces to the sequence {Xj, j ε 1} of independent random variables each distributed as X, and Sn becomes the ordinary partial sum 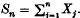 .
.
- Type
- Research Article
- Information
- Mathematical Proceedings of the Cambridge Philosophical Society , Volume 95 , Issue 1 , January 1984 , pp. 149 - 154
- Copyright
- Copyright © Cambridge Philosophical Society 1984
References
REFERENCES
- 7
- Cited by


