Hostname: page-component-cd9895bd7-fscjk Total loading time: 0 Render date: 2024-12-27T05:58:13.835Z Has data issue: false hasContentIssue false
  • English
  • Français

Quasiconformality and hyperbolic skew

Part of: Geometric function theory Analysis on metric spaces

Published online by Cambridge University Press:  18 December 2020

COLLEEN ACKERMANN  and
ALASTAIR FLETCHER
COLLEEN ACKERMANN
Affiliation:
Montgomery College, Rockville Campus, 51 Mannakee Street, Rockville, MD20850, U.S.A. e-mail: [email protected]
ALASTAIR FLETCHER
Affiliation:
Department of Mathematical Sciences, Northern Illinois University, DeKalb, IL60115-2888, U.S.A. e-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

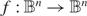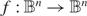We prove that if $f:\mathbb{B}^n \to \mathbb{B}^n$ , for n ≥ 2, is a homeomorphism with bounded skew over all equilateral hyperbolic triangles, then f is in fact quasiconformal. Conversely, we show that if $f:\mathbb{B}^n \to \mathbb{B}^n$ is quasiconformal then f is η-quasisymmetric in the hyperbolic metric, where η depends only on n and K. We obtain the same result for hyperbolic n-manifolds. 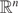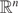Analogous results in $\mathbb{R}^n$ , and metric spaces that behave like $\mathbb{R}^n$ , are known, but as far as we are aware, these are the first such results in the hyperbolic setting, which is the natural metric to use on 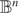$\mathbb{B}^n$ .

MSC classification

Primary: 30C62: Quasiconformal mappings in the plane
Secondary: 30L10: Quasiconformal mappings in metric spaces
Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 171 , Issue 3 , November 2021 , pp. 449 - 466
Copyright
© Cambridge Philosophical Society 2020

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

†

Supported by a grant from the Simons Foundation, #352034.

References

Ackermann, C., Haïssinsky, P. and Hinkkanen, A.. Equilateral triangle skew condition for quasiconformality Indiana Univ. Math. J. 68, No. 5 (2019), 1591–1608.CrossRefGoogle Scholar
Aramayona, J. and Haïssinsky, P.. A characterisation of plane quasiconformal maps using triangles, Publ. Mat. 52 (2008), no. 2, 459–471.CrossRefGoogle Scholar
Beardon, A. F. and Minda, D.. The hyperbolic metric and geometric function theory, Quasiconformal mappings and their applications (Narosa, New Delhi, 2007), 9–56.Google Scholar
Cao, C., Fletcher, A. and Ye, Z.. Epicycloids and Blaschke products. J. Difference Equ. Appl. 23, no.9 (2017), 1584–1596.Google Scholar
Fletcher, A. and Markovic, V.. Quasiconformal Mappings and Teichmüller Theory (OUP, 2007).Google Scholar
Fletcher, A. and Nicks, D. A.. Superattracting fixed points of quasiregular mappings. Ergodic Theory Dynam Systems, 36, no.3, (2016), 781–793.CrossRefGoogle Scholar
Gehring, F. W.. Rings and quasiconformal mappings in space. Trans. Amer. Math. Soc. 103 (1962), 353–393.CrossRefGoogle Scholar
Gehring, F. W. and Osgood, B. G.. Uniform domains and the quasi-hyperbolic metric. J. Anal. Math. 36 (1979), 50–74.CrossRefGoogle Scholar
Heinonen, J.. Lectures on Analysis on Metric Spaces (Springer-Verlag, 2001).CrossRefGoogle Scholar
Heinonen, J. and Koskela, P.. Quasiconformal maps in metric spaces with controlled geometry. Acta. Math. 181 (1998), 1–61.CrossRefGoogle Scholar
Hubbard, J. H.. Teichmüller Theory and Applications to Geometry, Topology and Dynamics, Volume 1: Teichmüller Theory (Matrix Editions, Ithaca, NY, 2006).Google Scholar
Li, Z.. Locally quasiconformal mappings and the Dirichlet problem of degenerate elliptic equations. Complex Var. Theory Appl. 23 (1993), 231–247.Google Scholar
Ratcliffe, J. G.. Foundations of hyperbolic manifolds, GTM 49 (Springer, 2006).Google Scholar
Rickman, S.. Quasiregular Mappings (Springer-Verlag, 1993).CrossRefGoogle Scholar
Trotsenko, D. A. and Väisälä, J.. Upper sets and quasisymmetric maps. Ann. Acad. Sci. Fenn. 24, no. 2 (1999), 465–488.Google Scholar
Väisälä, J.. Quasisymmetric embeddings in Euclidean spaces. Trans. Amer. Math. Soc. 264 (1981), no. 1, 191-204.CrossRefGoogle Scholar
Vuorinen, M.. Conformal Geometry and Quasiregular Mappings (Springer-Verlag, 1988).CrossRefGoogle Scholar
Vuorinen, M.. Conformal invariants and quasiregular mappings. J. Anal. Math. 45 (1985), 69–115.CrossRefGoogle Scholar