Hostname: page-component-586b7cd67f-g8jcs Total loading time: 0 Render date: 2024-11-26T12:46:17.527Z Has data issue: false hasContentIssue false
  • English
  • Français

Primitive divisors in arithmetic dynamics

Published online by Cambridge University Press:  01 March 2009

PATRICK INGRAM  and
JOSEPH H. SILVERMAN
PATRICK INGRAM
Affiliation:
Department of Mathematics, University of Toronto, Toronto, Ontario M5S 2E4, Canada. e-mail: [email protected]
JOSEPH H. SILVERMAN
Affiliation:
Mathematics Department, Box 1917 Brown University, Providence, RI 02912, U.S.A. e-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

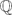Let ϕ(z) ∈ (z) be a rational function of degree d ≥ 2 with ϕ(0) = 0 and such that ϕ does not vanish to order d at 0. Let α ∈ 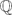have infinite orbit under iteration of ϕ and write ϕn(α) = An/Bn as a fraction in lowest terms. We prove that for all but finitely many n ≥ 0, the numerator An has a primitive divisor, i.e., there is a prime p such that p | An and p ∤ Ai for all i < n. More generally, we prove an analogous result when ϕ is defined over a number field and 0 is a preperiodic point for ϕ.

Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 146 , Issue 2 , March 2009 , pp. 289 - 302
Copyright
Copyright © Cambridge Philosophical Society 2008

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1]Arif, S. A. and Abu Muriefah, F. S.On the Diophantine equation x 2+q 2k+1 = y n. J. Number Theory 95 (1) (2002), 95–100.CrossRefGoogle Scholar
[2]Baker, M. A finiteness theorem for canonical heights attached to rational maps over function fields (2005) ArXiv:math.NT/0601046.Google Scholar
[3]Bang, A. S.Taltheoretiske Undersogelser. Tidsskrift Mat. 4 (5) (1886), 70–80, 130–137.Google Scholar
[4]Benedetto, R. L.Examples of wandering domains in p-adic polynomial dynamics. C. R. Math. Acad. Sci. Paris 335 (7) (2002), 615–620.Google Scholar
[5]Benedetto, R. L.Heights and preperiodic points of polynomials over function fields. Int. Math. Res. Not. 62 (2005), 3855–3866.CrossRefGoogle Scholar
[6]Benedetto, R. L.Wandering domains in non-Archimedean polynomial dynamics. Bull. London Math. Soc. 38 (6) (2006), 937–950.CrossRefGoogle Scholar
[7]Bilu, Y., Hanrot, G. and Voutier, P. M.Existence of primitive divisors of Lucas and Lehmer numbers. J. Reine Angew. Math. 539 (2001) 75–122. With an appendix by M. Mignotte.Google Scholar
[8]Birkhoff, G. D. and Vandiver, H. S.On the integral divisors of a n−b n. Ann. of Math. (2) 5 (4) (1904), 173–180.CrossRefGoogle Scholar
[9]Call, G. S. and Silverman, J. H.Canonical heights on varieties with morphisms. Compositio Math. 89 (2) (1993), 163–205.Google Scholar
[10]Carmichael, R. D.On the numerical factors of the arithmetic forms αn ± βn. Ann. of Math. (2) 15 (1–4) (1913/14), 30–48.CrossRefGoogle Scholar
[11]Everest, G., Mclaren, G. and Ward, T.Primitive divisors of elliptic divisibility sequences. J. Number Theory 118 (1) (2006), 71–89.CrossRefGoogle Scholar
[12]Flatters, A. Arithmetic dynamics of rational maps. preliminary manuscript (July 9, 2007).Google Scholar
[13]Ghioca, D. and Tucker, T. Equidistribution and integral points for Drinfeld modules (2006). ArXiv:math.NT.0609120.Google Scholar
[14]Hindry, M. and Silverman, J. H. Diophantine Geometry: An Introduction, volume 201 of Graduate Texts in Mathematics (Springer-Verlag, 2000).Google Scholar
[15]Hsia, L.-C. On the reduction of a non-torsion point of a Drinfeld module (2006). Preprint, NSC 90-2115-M-008-016.Google Scholar
[16]Ingram, P.Elliptic divisibility sequences over certain curves. J. Number Theory 123 (2) (2007), 473–486.CrossRefGoogle Scholar
[17]Ingram, P. and Silverman, J. H. Uniform estimates for primitive divisors in elliptic divisibility sequences. Volume in honor of Serge Lang (Springer-Verlag), to appear.Google Scholar
[18]Jones, R. The density of prime divisors in the arithmetic dynamics of quadratic polynomials (2006). ArXiv:math.NT/0612415.Google Scholar
[19]Lang, S.Fundamentals of Diophantine Geometry (Springer-Verlag, 1983).CrossRefGoogle Scholar
[20]Odoni, R. W. K.The Galois theory of iterates and composites of polynomials. Proc. London Math. Soc. (3) 51 (3) (1985), 385–414.CrossRefGoogle Scholar
[21]Odoni, R. W. K.On the prime divisors of the sequence w n+1 = 1+w 1⋅⋅⋅w n. J. London Math. Soc. (2) 32 (1) (1985), 1–11.CrossRefGoogle Scholar
[22]Poonen, B.Using elliptic curves of rank one towards the undecidability of Hilbert's tenth problem over rings of algebraic integers. In Algorithmic number theory (Sydney, 2002), Lecture Notes in Comput. Sci. vol. 2369 (Springer, 2002), pp 33–42.CrossRefGoogle Scholar
[23]Postnikova, L. P. and Schinzel, A.Primitive divisors of the expression a n−b n in algebraic number fields. Mat. Sb. (N.S.) 75 (117) (1968), 171–177.Google Scholar
[24]Praeger, C. E. Primitive prime divisor elements in finite classical groups. In Groups St. Andrews 1997 in Bath, II. London Math. Soc. Lecture Note Ser. vol. 261 (Cambridge University Press, 1999), pp 605–623.Google Scholar
[25]Rice, B.Primitive prime divisors in polynomial arithmetic dynamics. Integers (electronic), 7:A26 (2007), 16 pages.Google Scholar
[26]Schinzel, A.Primitive divisors of the expression A n−B n in algebraic number fields. J. Reine Angew. Math. 268/269 (1974), 27–33.Google Scholar
[27]Shparlinskiᄭ, I. E.The number of different prime divisors of recurrent sequences. Mat. Zametki 42 (4) (1987), 494–507, 622.Google Scholar
[28]Silverman, J. H.Wieferich's criterion and the abc-conjecture. J. Number Theory 30 (2) (1988), 226–237.CrossRefGoogle Scholar
[29]Silverman, J. H.The Arithmetic of Elliptic Curves. Graduate Texts in Mathematics, vol. 106 (Springer-Verlag, 1992).Google Scholar
[30]Silverman, J. H.Integer points, Diophantine approximation, and iteration of rational maps. Duke Math. J. 71 (3) (1993), 793–829.CrossRefGoogle Scholar
[31]Silverman, J. H.The Arithmetic of Dynamical Systems. Graduate Texts in Mathematics, vol. 241 (Springer-Verlag, 2007).Google Scholar
[32]Ward, M.Memoir on elliptic divisibility sequences. Amer. J. Math. 70 (1948), 31–74.CrossRefGoogle Scholar
[33]Zsigmondy, K.Zur Theorie der Potenzreste. Monatsh. Math. Phys. 3 (1) (1892), 265–284.CrossRefGoogle Scholar