Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Kallenberg, Olav
1976.
On the structure of stationary flat processes.
Zeitschrift f�r Wahrscheinlichkeitstheorie und Verwandte Gebiete,
Vol. 37,
Issue. 2,
p.
157.
Kallenberg, Olav
1977.
A counterexample to R. Davidson's conjecture on line processes.
Mathematical Proceedings of the Cambridge Philosophical Society,
Vol. 82,
Issue. 2,
p.
301.
Papangelou, F.
1977.
Conditional intensities of point processes: their application to Davidson's problem and to entropy.
Advances in Applied Probability,
Vol. 9,
Issue. 3,
p.
434.
Papangelou, F.
1977.
Conditional intensities of point processes: their application to Davidson's problem and to entropy.
Advances in Applied Probability,
Vol. 9,
Issue. 3,
p.
434.
Kallenberg, Olav
1978.
On conditional intensities of point processes.
Zeitschrift f�r Wahrscheinlichkeitstheorie und Verwandte Gebiete,
Vol. 41,
Issue. 3,
p.
205.
Kallenberg, Olav
1978.
On the asymptotic behavior of line processes and systems of non-interacting particles.
Zeitschrift f�r Wahrscheinlichkeitstheorie und Verwandte Gebiete,
Vol. 43,
Issue. 1,
p.
65.
Matthes, Klaus
Warmuth, Walter
and
Mecke, Joseph
1979.
Bemerkungen zu einer Arbeit von NGUYEN XUAN XANH und HANS ZESSIN.
Mathematische Nachrichten,
Vol. 88,
Issue. 1,
p.
117.
Kallenberg, Olav
1980.
On the structure of stationary flat processes. II.
Zeitschrift f�r Wahrscheinlichkeitstheorie und Verwandte Gebiete,
Vol. 52,
Issue. 2,
p.
127.
Kallenberg, Olav
1980.
Convergence of non-ergodic dynamical systems.
Zeitschrift f�r Wahrscheinlichkeitstheorie und Verwandte Gebiete,
Vol. 53,
Issue. 3,
p.
329.
Papangelou, F.
1980.
On the convergence of interacting particle systems to the poisson process.
Zeitschrift f�r Wahrscheinlichkeitstheorie und Verwandte Gebiete,
Vol. 52,
Issue. 3,
p.
235.
Fellous, A.
and
Granara, J.
1981.
Statistique des processus de Poisson stationnaires de sous-varietes lineaires affines.
Advances in Applied Probability,
Vol. 13,
Issue. 1,
p.
84.
Fellous, A.
and
Granara, J.
1981.
Statistique des processus de Poisson stationnaires de sous-varietes lineaires affines.
Advances in Applied Probability,
Vol. 13,
Issue. 1,
p.
84.
Kallenberg, Olav
1981.
On the structure of stationary flat processes. III.
Zeitschrift f�r Wahrscheinlichkeitstheorie und Verwandte Gebiete,
Vol. 56,
Issue. 2,
p.
239.
Kallenberg, Olav
2007.
Invariant measures and disintegrations with applications to Palm and related kernels.
Probability Theory and Related Fields,
Vol. 139,
Issue. 1-2,
p.
285.
Zessin, Hans
2009.
Der Papangelou Prozess.
Journal of Contemporary Mathematical Analysis,
Vol. 44,
Issue. 1,
p.
36.
Kallenberg, Olav
2014.
Stationary and invariant densities and disintegration kernels.
Probability Theory and Related Fields,
Vol. 160,
Issue. 3-4,
p.
567.
Rafler, Mathias
and
Zessin, Hans
2015.
The Logical Postulates of Böge, Carnap and Johnson in the Context of Papangelou Processes.
Journal of Theoretical Probability,
Vol. 28,
Issue. 4,
p.
1431.
Nehring, Benjamin
Rafler, Mathias
and
Zessin, Hans
2016.
Splitting‐characterizations of the Papangelou process.
Mathematische Nachrichten,
Vol. 289,
Issue. 1,
p.
85.
2020.
Poisson Line Cox Process.
Poghosyan, S.
and
Zessin, H.
2021.
Characterization and Uniqueness of Gibbs Processes by Means of Kirkwood–Salsburg Equations.
Lobachevskii Journal of Mathematics,
Vol. 42,
Issue. 10,
p.
2427.
 be the space of k-dimensional affine subspaces of Rn (to be called k-flats below), equipped with its natural topology ((14), p. 132).
be the space of k-dimensional affine subspaces of Rn (to be called k-flats below), equipped with its natural topology ((14), p. 132). 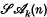 is a topological homogeneous space under the action of the group
is a topological homogeneous space under the action of the group 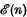 of Euclidean displacements in Rn and our purpose is to give conditions under which a stochastic point process on
of Euclidean displacements in Rn and our purpose is to give conditions under which a stochastic point process on 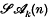 , stationary under
, stationary under 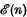 , is a doubly stochastic Poisson process. The results will be seen to apply equally well to abstract spaces sharing some of the structural properties of
, is a doubly stochastic Poisson process. The results will be seen to apply equally well to abstract spaces sharing some of the structural properties of 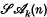 .
.

