Hostname: page-component-586b7cd67f-2plfb Total loading time: 0 Render date: 2024-11-27T01:34:54.072Z Has data issue: false hasContentIssue false
  • English
  • Français

Ordered orbits of the shift, square roots, and the devil's staircase

Published online by Cambridge University Press:  24 October 2008

Shaun Bullett  and
Pierrette Sentenac
Shaun Bullett
Affiliation:
School of Mathematical Sciences, Queen Mary and Westfield College, Mile End Road, LondonE1 4NS
Pierrette Sentenac
Affiliation:
Mathématique, Bâtiment 425, Université de Paris-Sud, 91405 Orsay, France
Get access Rights & Permissions [Opens in a new window]

Abstract

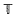An orbit of the shift σ: t ↦ 2t on the circle = ℝ/ℤ is ordered if and only if it is contained in a semi-circle Cμ = [μ, μ+½]. We investigate the ‘devil's staircase’ associating to each μ ε 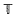the rotation number ν of the unique minimal closed σ-invariant set contained in Cμ; we present algorithms for μ in terms of ν, and we prove (after Douady) that if ν is irrational then μ is transcendental. We apply some of this analysis to questions concerning the square root map, and mode-locking for families of circle maps, we generalize our algorithms to orbits of the shift having ‘sequences of rotation numbers’, and we conclude with a characterization of all orders of points around 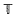realizable by orbits of σ.

Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 115 , Issue 3 , May 1994 , pp. 451 - 481
Copyright
Copyright © Cambridge Philosophical Society 1994

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1]Arnol'd, V. I.. Small denominators. I. Mapping the circle onto itself. Akad. Nauk. SSSR, Ser. Math. 25 (1961), 21–86, and Geometrical methods in the theory of ordinary differential equations (Springer-Verlag, 1983).Google Scholar
[2]Atela, P.. Bifurcations of dynamic rays in complex polynomials of degree two. Erg. Th. Dyn. Syst. 12 (1992), 401–423.CrossRefGoogle Scholar
[3]Bandt, C. and Keller, K.. Symbolic dynamics for angle-doubling on the circle. I. The topology of locally connected Julia sets. In Lecture Notes in Mathematics 1514 (Springer Verlag, 1992), 1–23.Google Scholar
[4]Bandt, C. and Keller, K.. Symbolic dynamics for angle-doubling on the circle. II. Symbolic dynamics of the abstract Mandelbrot set, preprint.Google Scholar
[5]Bandt, C. and Keller, K.. Symbolic dynamics for angle-doubling on the circle. III. Sturmian sequences and the quadratic map, preprint.Google Scholar
[6]Branner, B.. The Mandelbrot set. In Proceedings of Symposia in Applied Mathematics 39 (1989) (AMS Providence, Rhode Island).Google Scholar
[7]Douady, A.. Algorithm for computing angles in the Mandelbrot set. In Chaotic Dynamics and Fractals (Academic Press, 1986).Google Scholar
[8]Douady, A. and Hubbard, J. H.. Itération des polynomes quadratiques complexes. C.R. Acad. Sci. Paris, t294, Seri. I (1982), 123–126.Google Scholar
[9]Douady, A. and Hubbard, J. H.. Etude dynamique des polynomes complexes. (Publ. Math. Orsay I 1984, II, 1985).Google Scholar
[10]Douady, A. and Hubbard, J. H.. On the dynamics of polynomial-like mappings. Ann. Sci. Ec. Norm. Sup. (Paris) 18 (1985), 287–343.Google Scholar
[11]Gambaudo, J. M., Lanford, O. and Tresser, C.. Dynamique symbolique des rotations. C.R. Acad. Sci. Paris, t299 (1984), 823–825.Google Scholar
[12]Glendinning, P. A. and Sparrow, C. T.. Prime and renormalisable kneading invariants and the dynamics of expanding Lorenz maps. Physic 62D (1993), 22–50.Google Scholar
[13]Goldberg, L. R.. Fixed points of polynomial maps I. Ann Ec. Norm. Sup. 25 (1992), 679–685.CrossRefGoogle Scholar
[14]Goldberg, L. R. and Milnor, J.. Fixed points of polynomial maps II. Ann. Ec. Norm. Sup. 26 (1993), 51–98.Google Scholar
[15]Hubbard, J. H. and Sparrow, C. T.. The classification of topologically expansive Lorenz maps. Comm. Pure App. Math. 43 (1990), 431–444.CrossRefGoogle Scholar
[16]Lavaurs, P.. Une description combinatoire de l'involution definie par M sur les rationnels a denominateur impair. C.R. Acad. Sci. Paris, t303 (1986), 143–146.Google Scholar
[17]Morse, M. and Hedlund, G. A.. Symbolic Dynamics II. Sturmian Trajectories, Am. J. Math. 62 (1940), 1–42.CrossRefGoogle Scholar
[18]Roth, K. F.. Rational approximations to algebraic numbers. Mathematika 2 (1955), 1–20Google Scholar
Roth, K. F.. corrigendum, Mathematika 2 (1955), 168.CrossRefGoogle Scholar
[19]Veerman, J. J. P.. Symbolic dynamics and rotation numbers. Physica 134A (1986), 543–576.Google Scholar
[20]Veerman, J. J. P.. Symbolic dynamics of order-preserving orbits. Physica 29D (1987), 191–201.Google Scholar