Hostname: page-component-78c5997874-m6dg7 Total loading time: 0 Render date: 2024-11-05T21:44:27.018Z Has data issue: false hasContentIssue false
  • English
  • Français

On the product of three homogeneous linear forms

Published online by Cambridge University Press:  24 October 2008

J. H. H. Chalk  and
C. A. Rogers
J. H. H. Chalk
Affiliation:
Princeton University and The Institute for Advanced StudyPrinceton, N.J.
C. A. Rogers
Affiliation:
Princeton University and The Institute for Advanced StudyPrinceton, N.J.
Get access Rights & Permissions [Opens in a new window]

Extract

Let X denote the general point with coordinates (x1, x2, x3) in 3-dimensional space; and let P(X) be the function defined by

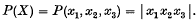
Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 47 , Issue 2 , April 1951 , pp. 251 - 259
Copyright
Copyright © Cambridge Philosophical Society 1951

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

* Davenport, H.Proc. London Math. Soc. (2), 44 (1938), 412–31CrossRefGoogle Scholar; for a simpler proof, see Davenport, H.J. London Math. Soc. 16 (1941), 98–101.CrossRefGoogle Scholar

† Davenport, H.Proc. Cambridge Phil. Soc. 39 (1943), 1–21.CrossRefGoogle Scholar

‡ Mahler, K.Proc. K. Ned. Akad. Wet. Amsterdam, 49 (1946), 331–43, 444–54, 524–32, 622–31Google Scholar (Theorem M, p. 527).

§ Davenport, H. and Rogers, C. A.Philos. Trans. Roy. Soc. A, 242 (1950), 311–44CrossRefGoogle Scholar, § 8.

∥ Loc. cit., Corollary to Theorem 12.

* J. London Math. Soc. 16 (1941), 98–101.Google Scholar

* Loc. cit. (1941).

* We use the method of Davenport, loc. cit. (1941).