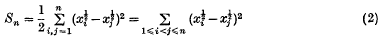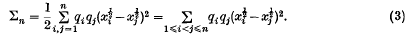Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Diananda, P. H.
1963.
On some inequalities of H. Kober: addendum.
Mathematical Proceedings of the Cambridge Philosophical Society,
Vol. 59,
Issue. 4,
p.
837.
Dinghas, Alexander
1968.
Superadditive Funktionale, Identit�ten und Ungleichungen der elementaren Analysis.
Mathematische Annalen,
Vol. 178,
Issue. 4,
p.
315.
Mitrinović, Dragoslav S.
1970.
Analytic Inequalities.
p.
27.
Bullen, P. S.
1971.
Rado's inequality.
Aequationes mathematicae,
Vol. 6,
Issue. 2-3,
p.
149.
Alzer, H.
1992.
Refinement of an inequality of H. Kober.
Periodica Mathematica Hungarica,
Vol. 25,
Issue. 3,
p.
269.
Gao, Peng
and
Qi, Feng
2009.
On an Inequality of Diananda—Part IV.
International Journal of Mathematics and Mathematical Sciences,
Vol. 2009,
Issue. 1,
Dragomir, Silvestru Sever
2023.
Determinant Inequalities for Positive Definite Matrices Via Diananda’s Result for Arithmetic and Geometric Weighted Means.
Annals of West University of Timisoara - Mathematics and Computer Science,
Vol. 59,
Issue. 1,
p.
21.