Hostname: page-component-586b7cd67f-g8jcs Total loading time: 0 Render date: 2024-11-29T18:15:57.206Z Has data issue: false hasContentIssue false
  • English
  • Français

On complex line arrangements and their boundary manifolds

Published online by Cambridge University Press:  25 May 2015

V. FLORENS ,
B. GUERVILLE-BALLÉ  and
M.A. MARCO-BUZUNARIZ
V. FLORENS
Affiliation:
LMA, UMR CNRS 5142, Université de Pau et des Pays de l'Adour, 64000 Pau, France. e-mail: [email protected]
B. GUERVILLE-BALLÉ
Affiliation:
IJF, UMR 5582 CNRS-UJF, Université Grenoble Alpes, 38 000 Grenoble, France. e-mail: [email protected]
M.A. MARCO-BUZUNARIZ
Affiliation:
ICMAT: CSIC-Complutense-Autonoma-Carlos III, Departamento de Algebra, Facultad de CC. Matematicas - Plaza de las Ciencias, 3, 28040 Madrid, Spain. e-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Let 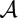${\mathcal A}$ be a line arrangement in the complex projective plane 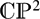$\mathds{C}\mathds{P}^2$. We define and describe the inclusion map of the boundary manifold, the boundary of a closed regular neighbourhood of 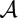${\mathcal A}$, in the exterior of the arrangement. We obtain two explicit descriptions of the map induced on the fundamental groups. These computations provide a new minimal presentation of the fundamental group of the complement.

Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 159 , Issue 2 , September 2015 , pp. 189 - 205
Copyright
Copyright © Cambridge Philosophical Society 2015 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1] Artal, E. Topology of arrangements and position of singularities. Annales de la fac. des sciences de Toulouse, to appear. (2014).Google Scholar
[2] Artal, E., Carmona, J., Cogolludo–Agustín, J.I. and Marco, M.A. Topology and combinatorics of real line arrangements. Compos. Math. 141 6 (2005), 1578–1588.Google Scholar
[3] Artal, E., Carmona, J., Cogolludo–Agustín, J.I. and Marco, M.A. Invariants of combinatorial line arrangements and Rybnikov's example. In Singularity theory and its applications, Izumiya, S., Ishikawa, G., Tokunaga, H., Shimada, I. and Sano, T., Eds. Adv. Stud. Pure Math. vol. 43 (Mathematical Society of Japan, Tokyo, 2007).Google Scholar
[4] Artal, E., Florens, V. and Guerville–Ballé, B. A topological invariant of line arrangements arXiv:1407.3387 (2014).Google Scholar
[5] Arvola, W. The fundamental group of the complement of an arrangement of complex hyperplanes. Topology 31 4 (1992), 757–765.Google Scholar
[6] Cohen, D. and Suciu, A. The boundary manifold of a complex line arrangement. In Groups, Homotopy and Configuration Spaces. Geom. Topol. Monogr. vol. 13 (Geom. Topol. Publ., Coventry, 2008), pp. 105–146.Google Scholar
[7] Guerville–Ballé, B. Topological invariants of line arrangements. PhD. thesis. Université de Pau et des Pays de l'Adour and Universidäd de Zaragoza (2013).Google Scholar
[8] Guerville–Ballé, B. An arithmetic Zariski 4-tuple of twelve lines, arXiv:1411.2300 (2014).Google Scholar
[9] Hironaka, E. Boundary manifolds of line arrangements. Math. Ann. 319 1 (2001), 17–32.Google Scholar
[10] Libgober, A. On the homotopy type of the complement to plane algebraic curves. J. Reine Angew. Math. 367 (1986), 103–114.Google Scholar
[11] MacLane, S. Some interpretations of abstract linear dependence in terms of projective geometry. Amer. J. Math. 58 1 (1936), 236–240.Google Scholar
[12] Neumann, W. A calculus for plumbing applied to the topology of complex surface singularities and degenerating complex curves. Trans. Amer. Math. Soc. 268 2 (1981), 299–344.Google Scholar
[13] Orlik, P. and Terao, H. Arrangements of Hyperplanes. Grundlehren Math. Wiss. vol. 300 (Springer-Verlag, Berlin, 1992).Google Scholar
[14] Rybnikov, G. On the fundamental group of the complement of a complex hyperplane arrangement. Preprint available at arXiv:math.AG/9805056 (1998).Google Scholar
[15] Waldhausen, F. Eine Klasse von 3-dimensionalen Mannigfaltigkeiten. I, II. Invent. Math. 3 (1967), 308–333; ibid. 4 (1967), 87–117.Google Scholar
[16] Westlund, E. The boundary manifold of an arrangement. PhD. thesis. University of Wisconsin Madison (1997).Google Scholar