Published online by Cambridge University Press: 24 October 2008
1. The plane quartic curves which pass through twelve fixed points g, of which no three lie on a straight line, no six on a conic and no ten on a cubic, form a net of quartics represented by the equation
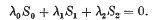
* See Küpper, C., “Über geometrische Netze” [Abh. d. k. bōhm. Gesell. d. Wiss., Prag, (7) I (1886)].Google Scholar The existence of such nets affords an example of the exceptions pointed out by Bacharach, [Math. Ann. XXVI (1886), 275]CrossRefGoogle Scholar to a theorem of Cayley's [Papers, I, 25] that a curve of order p=m+n-γ passing through
of the points of intersection of two curves of orders m and n passes also through the remaining
. This no longer holds if these
points lie on a curve of order γ -3. In our case m=n=γ=4. This example has been given for many years by Mr Richmond in his lectures on Plane Algebraic Geometry at Cambridge.
† See White, F. P., Proc. Camb. Phil. Soc. XXI (1922), 216.Google Scholar
* Projecting from P on to a hyperplane, we get a quintic surface in ordinary space with a double twisted cubic curve; this surface was investigated years ago by Clebsch, [Math. Ann. 1 (1869), 284]Google Scholar; the hyperelliptic curve is the representation of the double curve.