The nuclearity of operators generated by Hölder continuous kernels
Published online by Cambridge University Press: 24 October 2008
Extract
Introduction. The space Cp is the class of operators on a Hilbert space for which the norm ∥K∥p = [trace (KK*)P/2]/p is finite. Equivalently, a compact operator is in Cp if
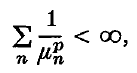
where the μn are the so-called ‘singular values’ of K (characteristic values of the non-negative compact operator [K] ≡ (KK*)½). The case p = 2 gives the familiar class of Hilbert–Schmidt operators, while C1 is the collection of trace-class or nuclear operators considered by Schatten(12), Lidskii(11), and Gohberg and Krein(7), among others.
- Type
- Research Article
- Information
- Mathematical Proceedings of the Cambridge Philosophical Society , Volume 75 , Issue 3 , May 1974 , pp. 351 - 356
- Copyright
- Copyright © Cambridge Philosophical Society 1974
References
REFERENCES
- 6
- Cited by


