No CrossRef data available.
Published online by Cambridge University Press: 24 October 2008
Let V be a smooth complex algebraic threefold with k(V) = 0. Consider the space of holomorphic 2-forms on V, and let s denote the rank of the subsheaf of 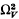 generated by these global forms. It was conjectured by Uenoin[8] that
generated by these global forms. It was conjectured by Uenoin[8] that 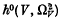 ≤ 3-here as always, hi denotes the dimension of the corresponding cohomology group Hi.
≤ 3-here as always, hi denotes the dimension of the corresponding cohomology group Hi.