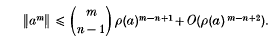Hostname: page-component-78c5997874-94fs2 Total loading time: 0 Render date: 2024-11-06T12:47:46.326Z Has data issue: false hasContentIssue false
  • English
  • Français

Norm and spectral radius for algebraic elements of a Banach algebra

Published online by Cambridge University Press:  24 October 2008

N. J. Young
N. J. Young
Affiliation:
University of Glasgow
Get access Rights & Permissions [Opens in a new window]

Extract

The purpose of this note is to show that, for any algebraic element a of a Banach algebra and certain analytic functions f, one can give an upper bound for ‖f(a)‖ in terms of ‖a‖ and the spectral radius ρ(a) of a. To illustrate the nature of the result, consider the norms of powers of an element a of unit norm. In general, the spectral radius formula

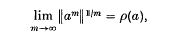
contains all that can be said (that is, the limit ρ(a) can be approached arbitrarily slowly). If we have the additional information that a is algebraic of degree n we can say a good deal more. In the case of a C*-algebra we have the neat result that, if ‖a‖ ≤ 1,

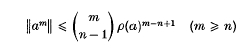
(see Theorem 2), while for a general Banach algebra we have at least

Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 88 , Issue 1 , July 1980 , pp. 129 - 133
Copyright
Copyright © Cambridge Philosophical Society 1980

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

(1)Dostál, Z. Norms of iterates and the spectral radius of matrices. (To appear.)Google Scholar
(2)Dostál, Z.Polynomials of the eigenvalues and powers of matrices. Comment. Math. Univ. Carolinae 19 (1978), 459–469.Google Scholar
(3)Pták, V.Spectral radius, norms of iterates and the critical exponent. Linear Algebra and Appl. 1 (1968), 245–260.CrossRefGoogle Scholar
(4)Pták, V.An infinite companion matrix. Comment. Math. Univ. Carolinae 19 (1978), 447–458.Google Scholar
(5)Young, N. J.Norms of matrix powers. Comment. Math. Univ. Carolinae 19 (1978), 415–430.Google Scholar
(6)Young, N. J. The rate of convergence of a matrix power series. Letters in Linear Algebra. (To appear.)Google Scholar
(7)Nagy, B.Sz. Sur la norme des fonctions de certains opérateurs. Acta Math. Acad. Sci. Hungar. 20 (1969), 331–334.CrossRefGoogle Scholar
(8)Sarason, D.Generalized interpolation in H ∞. Trans. Amer. Math. Soc. 127 (1967), 179–203.Google Scholar