The Markov branching process with density-independent catastrophes I. Behaviour of extinction probabilities
Published online by Cambridge University Press: 24 October 2008
Extract
Let (Xt: t ≥ 0) be the Markov branching process (MBP) with a density independent catastrophe component. It is denned to be the Feller process on the non-negative integers having the generator
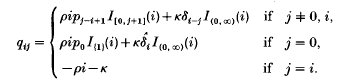
Here {pj} is the offspring distribution which satisfies p1 = 0 and p0 < 1, ρ is the per capita birth rate, κ is the rate of occurrence of catastrophe events, {δj: j ≥ 0} is the decrement distribution and 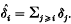 . Thus Xt can be interpreted as the size of a population in which individuals reproduce according to the rules of a MBP – see Athreya and Ney[1], chap, III – and where there is an external and independent Poisson process of catastrophe events, κ per unit time, and if j < i each such event reduces the population size by j with probability δj. Usually we assume that δ0 = 0 on the basis that a catastrophe always reduces the population size. Let f(s) = Σpjsj and assume that
. Thus Xt can be interpreted as the size of a population in which individuals reproduce according to the rules of a MBP – see Athreya and Ney[1], chap, III – and where there is an external and independent Poisson process of catastrophe events, κ per unit time, and if j < i each such event reduces the population size by j with probability δj. Usually we assume that δ0 = 0 on the basis that a catastrophe always reduces the population size. Let f(s) = Σpjsj and assume that 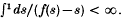 . This ensures that the MBP obtained by setting κ = 0 is regular ([1], p. 105) and hence (Xt) is the unique Markov process corresponding to the above generator when κ > 0.
. This ensures that the MBP obtained by setting κ = 0 is regular ([1], p. 105) and hence (Xt) is the unique Markov process corresponding to the above generator when κ > 0.
- Type
- Research Article
- Information
- Mathematical Proceedings of the Cambridge Philosophical Society , Volume 103 , Issue 2 , March 1988 , pp. 351 - 366
- Copyright
- Copyright © Cambridge Philosophical Society 1988
References
REFERENCES
- 9
- Cited by


