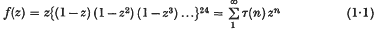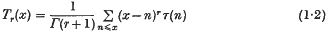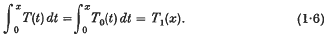Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Rankin, R. A.
1940.
Contributions to the theory of Ramanujan's function τ(n) and similar arithmetical functions.
Mathematical Proceedings of the Cambridge Philosophical Society,
Vol. 36,
Issue. 2,
p.
150.
Guinand, A. P.
1947.
Integral modular forms and summation formulae.
Mathematical Proceedings of the Cambridge Philosophical Society,
Vol. 43,
Issue. 1,
p.
127.
Pennington, W. B.
1951.
On the order of magnitude of Ramanujan's arithmetical function τ(n).
Mathematical Proceedings of the Cambridge Philosophical Society,
Vol. 47,
Issue. 4,
p.
668.
Chandrasekharan, K.
and
Narasimhan, Raghavan
1961.
On Hecke’s functional equation.
Bulletin of the American Mathematical Society,
Vol. 67,
Issue. 2,
p.
182.
Iwasaki, Koziro
1963.
Note on the modular forms.
Proceedings of the Japan Academy, Series A, Mathematical Sciences,
Vol. 39,
Issue. 6,
Berndt, Bruce C.
1969.
Arithmetical identities and Hecke's functional equation.
Proceedings of the Edinburgh Mathematical Society,
Vol. 16,
Issue. 3,
p.
221.
Berndt, Bruce C.
1975.
Identities involving the coefficients of a class of Dirichlet series. VII.
Transactions of the American Mathematical Society,
Vol. 201,
Issue. 0,
p.
247.
Hejhal, Dennis A.
1979.
A note on the Vorono� summation formula.
Monatshefte f�r Mathematik,
Vol. 87,
Issue. 1,
p.
1.
Fomenko, O. M.
1980.
Applications of the theory of modular forms to number theory.
Journal of Soviet Mathematics,
Vol. 14,
Issue. 4,
p.
1307.
Rankin, R. A.
1986.
Fourier coefficients of cusp forms.
Mathematical Proceedings of the Cambridge Philosophical Society,
Vol. 100,
Issue. 1,
p.
5.
Heath, Sister Ann M.
2016.
Dirichlet series with functional equations and arithmetical identities.
The Ramanujan Journal,
Vol. 41,
Issue. 1-3,
p.
115.
Chen, William Y. C.
Du, Julia Q. D.
and
Zhao, Jack C. D.
2019.
Finding Modular Functions for Ramanujan-Type Identities.
Annals of Combinatorics,
Vol. 23,
Issue. 3-4,
p.
613.
Roy, Arindam
Sahoo, Jagannath
and
Vatwani, Akshaa
2024.
Equivalence between the functional equation and Voronoï-type summation identities for a class of L-functions.
Proceedings of the Royal Society of Edinburgh: Section A Mathematics,
p.
1.