No CrossRef data available.
Explicit formulae connecting Hölder's, Cesàro's and another mean value
Published online by Cambridge University Press: 24 October 2008
Extract
1. Having given the terms sn of a sequence
then Hölder's means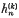 
are defined by
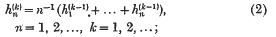
Cesàro's means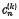 
are defined by
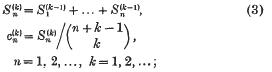
and a third kind, σ, of mean which will be used in this paper is defined by
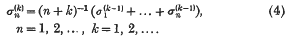
- Type
- Research Article
- Information
- Mathematical Proceedings of the Cambridge Philosophical Society , Volume 28 , Issue 1 , January 1932 , pp. 1 - 17
- Copyright
- Copyright © Cambridge Philosophical Society 1932
References
* Schur, I., Journal f. d. reine u. angew. Math., 151 (1921), 79–111.Google Scholar
* I. Schur, op. cit.
† Knopp, K., Math. Zeitschrift, 31 (1930), 97–127.CrossRefGoogle Scholar
‡ Schur, I., Math. Ann., 74 (1913), 447–458CrossRefGoogle Scholar; see Landau, E., Darstellung und Begründung u.s.w. (1916), § 5.Google Scholar
* Kienast, , “Extensions of Abel's theorem and its converses”, Proc. Camb. Phil. Soc. 19 (1920), 129–147Google Scholar.
* I am indebted to Prof. Hardy for the suggestion to consult in particular the paper of Ford.
† Ford, W. B., “On the relation between the sum-formulas of Hölder and Cesàro”, Amer. J. Math. 32 (1910), 315–326.CrossRefGoogle Scholar
‡ Kienast, , Proc. Camb. Phil. Soc. 20 (1921), 74–82.Google Scholar
* Kienast, , Proc. Camb. Phil. Soc. 20 (1921), 74–82.Google Scholar
* Schlömilch, , Compendium d. höheren Analysis, 2 (1895), 12, 27.Google Scholar
* Kienast, , Proc. Camb. Phil. Soc. 20 (1921), 74–82.Google Scholar