Published online by Cambridge University Press: 24 October 2008
If five spheres in 3-space are such that each pair is inclined at the same non-zero angle θ, then  where b1, …, b5 (the ‘bends’ (1) of the spheres) are the reciprocals of their radii. To prove this result, establish a system of rectangular cartesian coordinates (x, y, z) and let the spheres have centres (xi, yi, zi) and radii
where b1, …, b5 (the ‘bends’ (1) of the spheres) are the reciprocals of their radii. To prove this result, establish a system of rectangular cartesian coordinates (x, y, z) and let the spheres have centres (xi, yi, zi) and radii 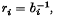 , where i = 1,…, 5. Then for x5, y5, z5, r5 we have the equations
, where i = 1,…, 5. Then for x5, y5, z5, r5 we have the equations 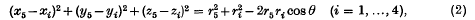 which, on subtraction, yield three linear equations and one quadratic equation. Solving the three linear equations for x5, y5, z5 and substituting, we see that the required relation is algebraic (indeed quadratic) in r5 and hence in b5. Since it is also symmetric in b1,…, b5, it follows that it can be expressed as a polynomial relation in the elementary symmetric functions p1, …, p5 in b5, …, b5.
which, on subtraction, yield three linear equations and one quadratic equation. Solving the three linear equations for x5, y5, z5 and substituting, we see that the required relation is algebraic (indeed quadratic) in r5 and hence in b5. Since it is also symmetric in b1,…, b5, it follows that it can be expressed as a polynomial relation in the elementary symmetric functions p1, …, p5 in b5, …, b5.