Published online by Cambridge University Press: 08 July 2016
Under the Riemann Hypothesis, we connect the distribution of k-free numbers with the derivative of the Riemann zeta-function at nontrivial zeros of ζ(s). Moreover, with additional assumptions, we prove the existence of a limiting distribution of 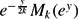 $e^{-\frac{y}{2k}}M_k(e^y)$ and study the tail of the limiting distribution, where
$e^{-\frac{y}{2k}}M_k(e^y)$ and study the tail of the limiting distribution, where 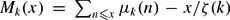 $M_k(x)=\sum_{n\leq x}\mu_k(n)-{x}/{\zeta(k)}$ and μk(n) is the characteristic function of k-free numbers. Finally, we make a conjecture about the maximum order of Mk(x) by heuristic analysis on the tail of the limiting distribution.
$M_k(x)=\sum_{n\leq x}\mu_k(n)-{x}/{\zeta(k)}$ and μk(n) is the characteristic function of k-free numbers. Finally, we make a conjecture about the maximum order of Mk(x) by heuristic analysis on the tail of the limiting distribution.