No CrossRef data available.
Published online by Cambridge University Press: 06 September 2023
Let 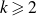 $k \geqslant 2$ be an integer. We prove that factorisation of integers into k parts follows the Dirichlet distribution
$k \geqslant 2$ be an integer. We prove that factorisation of integers into k parts follows the Dirichlet distribution 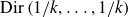 $\mathrm{Dir}\left({1}/{k},\ldots,{1}/{k}\right)$ by multidimensional contour integration, thereby generalising the Deshouillers–Dress–Tenenbaum (DDT) arcsine law on divisors where
$\mathrm{Dir}\left({1}/{k},\ldots,{1}/{k}\right)$ by multidimensional contour integration, thereby generalising the Deshouillers–Dress–Tenenbaum (DDT) arcsine law on divisors where 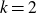 $k=2$. The same holds for factorisation of polynomials or permutations. Dirichlet distribution with arbitrary parameters can be modelled similarly.
$k=2$. The same holds for factorisation of polynomials or permutations. Dirichlet distribution with arbitrary parameters can be modelled similarly.