Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Kalton, N. J.
1975.
The Theory and Application of Differential Games.
p.
45.
Elliott, Robert J.
and
Friedman, Avner
1975.
A Note on Generalized Pursuit-Evasion Games.
SIAM Journal on Control,
Vol. 13,
Issue. 1,
p.
105.
Godefroy, G.
2014.
Obituary: Nigel John Kalton, 1946–2010.
Bulletin of the London Mathematical Society,
Vol. 46,
Issue. 6,
p.
1292.


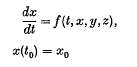
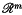 , with pay-off
, with pay-off