Article contents
The convolution metric dg
Published online by Cambridge University Press: 24 October 2008
Abstract
We introduce and study a new metric on  denned by
denned by
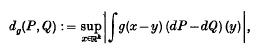
where  is the space of probability measures on ℝk and where g: ℝk→ is a probability density satisfying certain mild conditions. The metric dg, relatively easy to compute, is shown to have useful and interesting properties not enjoyed by some other metrics on
is the space of probability measures on ℝk and where g: ℝk→ is a probability density satisfying certain mild conditions. The metric dg, relatively easy to compute, is shown to have useful and interesting properties not enjoyed by some other metrics on  . In particular, letting pn denote the nth empirical measure for P, it is shown that under appropriate conditions
. In particular, letting pn denote the nth empirical measure for P, it is shown that under appropriate conditions  satisfies a compact law of the iterated logarithm, converges in probability to the supremum of a Gaussian process, and has a useful stochastic integral representation.
satisfies a compact law of the iterated logarithm, converges in probability to the supremum of a Gaussian process, and has a useful stochastic integral representation.
- Type
- Research Article
- Information
- Mathematical Proceedings of the Cambridge Philosophical Society , Volume 98 , Issue 3 , November 1985 , pp. 533 - 540
- Copyright
- Copyright © Cambridge Philosophical Society 1985
References
REFERENCES
- 7
- Cited by


