Research Article
On the Development of Mathematical Analysis and its Relations to Some other Sciences*
-
- Published online by Cambridge University Press:
- 03 November 2016, pp. 193-201
-
- Article
- Export citation
I.—Expansions of Trigonometrical Functions
-
- Published online by Cambridge University Press:
- 03 November 2016, pp. 202-206
-
- Article
- Export citation
II—Expansion of Functions in General
-
- Published online by Cambridge University Press:
- 03 November 2016, pp. 206-208
-
- Article
- Export citation
Other
Constructions with Straight-Edge and Dividers
-
- Published online by Cambridge University Press:
- 03 November 2016, pp. 209-211
-
- Article
- Export citation
Mathematical Notes
170. Negative quantities (a very elementary note)
-
- Published online by Cambridge University Press:
- 03 November 2016, pp. 211-212
-
- Article
- Export citation
171. On the normals from a point to a conicoid
-
- Published online by Cambridge University Press:
- 03 November 2016, p. 213
-
- Article
- Export citation
172. Three conics connected with a triangle
-
- Published online by Cambridge University Press:
- 03 November 2016, pp. 213-214
-
- Article
- Export citation
Review
Elements of Mechanics. Forty Lessons for Beginners in Engineering. By Mansfield Merriman, Professor of Civil Engineering in Lehigh University. (New York, Wiley & Sons.London, Chapman & Hall. 1905. pp. 172. $1.)
-
- Published online by Cambridge University Press:
- 03 November 2016, p. 215
-
- Article
- Export citation
Other
Errata
-
- Published online by Cambridge University Press:
- 03 November 2016, p. 215
-
- Article
- Export citation
Column for “Queries,” “Sale and Exchange,” “Wanted,” Etc
-
- Published online by Cambridge University Press:
- 03 November 2016, p. 216
-
- Article
- Export citation
Books Received
Books, Etc., Received
-
- Published online by Cambridge University Press:
- 03 November 2016, pp. 216-217
-
- Article
- Export citation
Front matter
MAG volume 3 issue 52 and Front matter
-
- Published online by Cambridge University Press:
- 03 November 2016, pp. f1-f2
-
- Article
-
- You have access
- Export citation
Back matter
MAG volume 3 issue 52 and Front matter
-
- Published online by Cambridge University Press:
- 03 November 2016, pp. b1-b2
-
- Article
-
- You have access
- Export citation

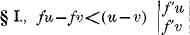 where
where 