Hostname: page-component-586b7cd67f-t7fkt Total loading time: 0 Render date: 2024-11-28T08:48:58.983Z Has data issue: false hasContentIssue false
  • English
  • Français

Repeated vector products

Published online by Cambridge University Press:  08 October 2020

A. F. Beardon  and
N. Lord
A. F. Beardon
Affiliation:
Centre for Mathematical Sciences, University of Cambridge, Wilberforce Rd., Cambridge CB3 0WB e-mail: [email protected]
N. Lord
Affiliation:
Tonbridge School, Tonbridge, Kent TN9 1JP e-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Extract

In [1] the second author observed that it is possible to have a binary operation * on a set X with the property that two different arrangements of brackets in a given combination x1 * … * xn of elements of X yield the same outcome for all choices of the xj. For example, for the operation of subtraction on the set of real numbers, we have

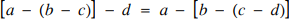$$\left[ {a - \left( {b - c} \right)} \right] - d = a - \left[ {b - \left( {c - d} \right)} \right]$$
for all real numbers a, b, c and d. The author then asked whether or not a similar example might hold for an n-fold vector product on three-dimensional Euclidean space3. We shall show here that no such example can exist; thus two different arrangements of brackets in a repeated vector product will, for some vectors, yield different answers.

Type
Articles
Information
The Mathematical Gazette , Volume 104 , Issue 561 , November 2020 , pp. 460 - 468
Check for updates
Copyright
© Mathematical Association 2020

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Lord, N. J., Non-associative operations, Math. Mag. 60 (1987) pp. 174–177.CrossRefGoogle Scholar
Leversha, G. and Rowland, D., Introduction to combinatorics, UK Mathematics Trust (2015) pp. 80–98.Google Scholar
Godement, R., Algebra, Kershaw Pub. Co., London, 1969.Google Scholar
Quadling, D. A., Q for quaternions, Math. Gaz. 63 (June 1979) pp. 98–110.CrossRefGoogle Scholar
Weston, J. D., Vectors as quaternions: A corner of linear algebra, Math. Gaz. 85 (March 2001) pp. 25–35.10.2307/3620466CrossRefGoogle Scholar
Pritchard, C., Tendril of the hop and of the vine, Part I, Math. Gaz. 82 (March 1998) pp. 26–36.10.2307/3620147CrossRefGoogle Scholar
Pritchard, C., Flaming swords and hermaphrodite monsters - Peter Guthrie Tait and the promotion of quaternions, Part II, Math. Gaz. 82 (July 1998) pp. 235–241.10.2307/3620406CrossRefGoogle Scholar