No CrossRef data available.
Article contents
Expressing harmonic sums as fractions
Published online by Cambridge University Press: 15 June 2017
Extract
As usual, write
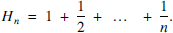
In [1], it is shown, by an elegant method, that for all n ⩾ 2, Hn is not an integer. The method can be traced back at least to [2, Exercise 251, p. 159]. Actually, rather more is shown: if Hn is expressed as a fraction an/bn in its lowest terms, then for all n ⩾ 2, bn is even. One way of looking at this is to say that once the term 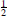 has entered the sum, the factor 2 persists in the denominator from then on. This suggests the following question:
has entered the sum, the factor 2 persists in the denominator from then on. This suggests the following question:
(Q1) for primes p ⩾ 3, is bn a multiple of p for all n ⩾ p?
and more generally:
(Q2) for any prime p, is bn a multiple of pm for all n ⩾ pm?
In other words, once 1/pm has entered the sum, does the factor pm persist in the denominator?
Let dn denote the lowest common multiple of 1, 2, … , n. This number can be described as follows: for each prime p ⩽ n, let mp be the largest integer m such that pm ⩽ n. Then 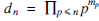 . Hence a positive answer to (Q2) would imply that bn simply equals dn.
. Hence a positive answer to (Q2) would imply that bn simply equals dn.
- Type
- Articles
- Information
- Copyright
- Copyright © Mathematical Association 2017


