Hostname: page-component-586b7cd67f-tf8b9 Total loading time: 0 Render date: 2024-11-24T20:50:26.898Z Has data issue: false hasContentIssue false
  • English
  • Français

The Exponential and Logarithmic Functions

Published online by Cambridge University Press:  03 November 2016

R. Cooper
Get access Rights & Permissions [Opens in a new window]

Extract

The usual method of developing the theory of the exponential and logarithmic functions is to take as a definition either

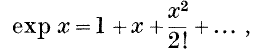
or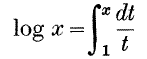

The first definition has the disadvantage that with it a good deal of the theory of series is needed. Neither development follows the lines on which the student first met the functions. A good deal could be said for the view that for most students the best type of accurate theory is one which fills up the gaps in methods which are already familiar The difficulties of taking

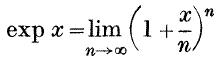
as a definition are well known.

Type
Research Article
Information
The Mathematical Gazette , Volume 20 , Issue 240 , October 1936 , pp. 258 - 263
Copyright
Copyright © Mathematical Association 1936

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

page no 258 note * See H. G. Forder, Foundations of Euclidean geometry (1927).

page no 258 note † See G. H. Hardy, Pure Mathematics, p. 139, or Hardy, Littlewood and Pól;ya, Inequalities, Th. 41, p. 39. These are referred to as P.M. and H.L.P. respectively.

page no 259 note * The obvious extension has “≤” in place of “<”, but this is enough for our purpose. For the extension with “<” see H.L.P., p. 41, or use methods suggested on p. 18 of H.L.P.

page no 259 note † We have used the rule

page no 260 note * In P.M. (5th ed., p. 387) these are based on a definition of The method here avoids the infinities of tan x and enables us to use Euler’s beautiful proof of the addition theorem.

page no 260 note † C(x) and finite because, if 0≤x≤1–2−n ,

page no 261 note * The verification of the derivatives is almost trivial except at multiples of ½Π, and here is much simpler than if our basis were tan−1 x, as we do not have to deal with the infinities of tan x.

page no 262 note * We have defined and use only the principal value of log z.

page no 262 note † The error term is the sum of a finite number of terms each of the form

O(|x| r |y| s ) where n + 1 ≤ r + s ≤ 2(n + 1).