Article contents
Closed form expressions for two harmonic continued fractions
Published online by Cambridge University Press: 16 October 2017
Extract
A continued fraction is an expression of the form
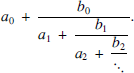
The expression can continue for ever, in which case it is called an infinite continued fraction, or it can stop after some term, when we call it a finite continued fraction. For irrational numbers, a continued fraction expansion often reveals beautiful number patterns which remain obscured in their decimal expansion. The interested reader is referred to [1] for a collection of many interesting continued fractions for famous mathematical constants.
- Type
- Articles
- Information
- Copyright
- Copyright © Mathematical Association 2017
References
- 2
- Cited by


