No CrossRef data available.
Published online by Cambridge University Press: 12 October 2022
It was known before Archimedes (287-212 BC) that the circumference of a circle was proportional to its diameter and that the area was proportional to the square of its radius. It was Archimedes who first supplied a rigorous proof that these two proportionality constants were the same, now called π [1]. He started with inscribed and circumscribed hexagons and increased the number of sides from 6 up to 96 by successively doubling it. His result was not a single value. In fact he generated five intervals each of which contained π. He calculated a lower bound from the inscribed polygon and an upper bound from the circumscribed polygon of 96 sides. This gave him the interval (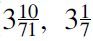 ) or (3.140845, 3.142857), which is less accurate than the interval bounded by half-perimeters of the inscribed and circumscribed 96-gons, which is (3.141031, 3.142714).
) or (3.140845, 3.142857), which is less accurate than the interval bounded by half-perimeters of the inscribed and circumscribed 96-gons, which is (3.141031, 3.142714).